Virial decomposition

virial series

The series on the right-hand side of the equation of state of a gas:

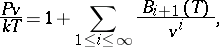where is the pressure, is the temperature, is the specific volume, and is the Boltzmann constant. The term of the series which contains the -th virial coefficient describes the deviation of the gas from ideal behaviour due to the interaction in groups of molecules. can be expressed in terms of irreducible repeated integrals :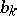

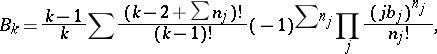summed over all natural numbers , , subject to the condition

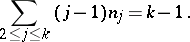In particular,

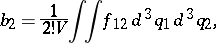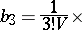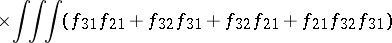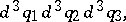where

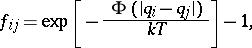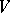is the volume of the gas, the integration extends over the total volume occupied by the gas, and is the interaction potential. There is a rule for writing down for any in terms of 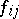. The expression obtained after simplification is:

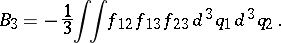In practice, only the first few virial coefficients can be calculated.

Power series in , with coefficients expressed in terms of , can be used to represent equilibrium correlation functions for particles; a corollary of this fact is that the equation of state can be obtained in a simple manner [3].

There exists a quantum-mechanical analogue of the virial decomposition.

References

[1]	J.E. Mayer, M. Goeppert-Mayer, "Statistical mechanics" , Wiley (1940)
[2]	R. Feynman, "Statistical mechanics" , M.I.T. (1972)
[3]	N.N. Bogolyubov, "Problems of a dynamical theory in statistical physics" , North-Holland (1962) (Translated from Russian)
[4]	G.E. Uhlenbeck, G.V. Ford, "Lectures in statistical mechanics" , Amer. Math. Soc. (1963)