Algebra with associative powers
A linear algebra 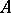 over a field
over a field 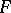 each element of which generates an associative subalgebra. The set of all algebras with associative powers over a given field
each element of which generates an associative subalgebra. The set of all algebras with associative powers over a given field  forms a variety of algebras which, if the characteristic of the field
forms a variety of algebras which, if the characteristic of the field 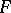 is zero, is defined by the system of identities
is zero, is defined by the system of identities
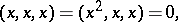 |
where 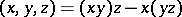 . If
. If  is an infinite field of prime characteristic
is an infinite field of prime characteristic 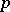 , then the variety of algebras with associative powers cannot be defined by any finite system of identities, but an independent, infinite system of identities which defines it is known [3]. If a commutative algebra
, then the variety of algebras with associative powers cannot be defined by any finite system of identities, but an independent, infinite system of identities which defines it is known [3]. If a commutative algebra 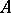 with associative powers of characteristic other than 2 has an idempotent
with associative powers of characteristic other than 2 has an idempotent 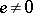 , then
, then 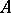 can be decomposed according to Peirce into a direct sum of vector subspaces:
can be decomposed according to Peirce into a direct sum of vector subspaces:
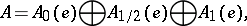 | (*) |
where 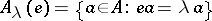 ,
, 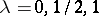 . Here
. Here  and
and  are subalgebras,
are subalgebras,  ,
, 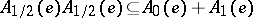 ,
, 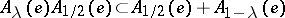 for
for  . The decomposition (*) plays a fundamental role in the structure theory of algebras with associative powers.
. The decomposition (*) plays a fundamental role in the structure theory of algebras with associative powers.
References
| [1] | A.A. Albert, "Power-associative rings" Trans. Amer. Math. Soc. , 64 (1948) pp. 552–593 |
| [2] | A.T. Gainov, "Identity relations for binary Lie rings" Uspekhi Mat. Nauk , 12 : 3 (1957) pp. 141–146 (In Russian) |
| [3] | A.T. Gainov, "Power-associative algebras over a finite-characteristic field" Algebra and Logic , 9 : 1 (1970) pp. 5–19 Algebra i Logika , 9 : 1 (1970) pp. 9–33 |
Comments
An algebra with associative powers is also called a power-associative algebra. The fact that the set of algebras with associative powers over a field of non-zero characteristic forms a variety defined by 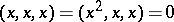 was proved in [a1].
was proved in [a1].
References
| [a1] | A.A. Albert, "On the power associativity of rings" Summa Brasiliensis Math. , 2 (1948) pp. 21–33 |
Algebra with associative powers. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Algebra_with_associative_powers&oldid=16404