Order relation

comparison of functions, 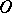- relations, asymptotic relations

A notion arising in studies on the behaviour of a function with respect to another function in a neighbourhood of some point (this point may be infinite).

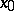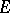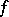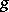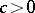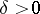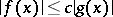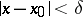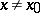Let be a limit point of a set . If for two functions and there exist constants and such that for , , then 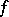is called a function which is bounded in comparison with 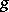in some deleted neighbourhood of 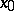, and this is written as

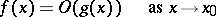(read "f is of the order of g" ); 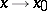means that the considered property holds only in some deleted neighbourhood of 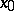. This definition can be naturally used when , .

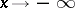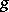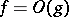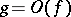If two functions and are such that and as 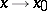, then they are called functions of the same order as 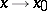. For instance, if two functions 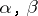are such that , 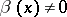if 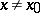and if the limit

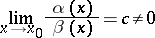exists, then they are of the same order as .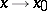

Two functions 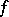and 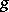are called equivalent (asymptotically equal) as 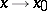(written as 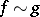) if in some neighbourhood of 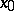, except maybe the point 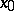itself, a function 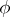is defined such that

(*)

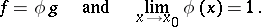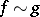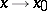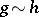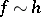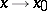The condition of equivalency of two functions is symmetric, i.e. if , then as , and transitive, i.e. if and , then as . If in some neighbourhood of the point 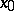the inequalities 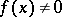, 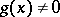hold for , then (*) is equivalent to any of the following conditions:

If 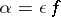where 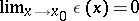, then is said to be an infinitely-small function with respect to 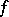, and one writes

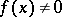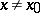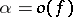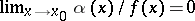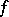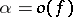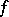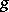(read "a is of lower order than f" ). If when , then if . If is an infinitely-small function for , one says that the function is an infinitely-small function of higher order than as . If and 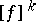are quantities of the same order, then one says that 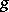is a quantity of order 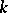with respect to 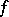. All formulas of the above type are called asymptotic estimates; they are especially interesting for infinitely-small and infinitely-large functions.

Examples: 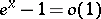(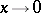); ; 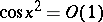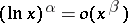(; 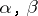any positive numbers); ().

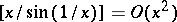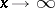Here are some properties of the symbols and :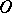

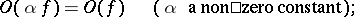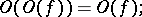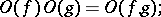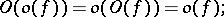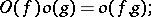if 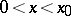and 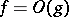, then

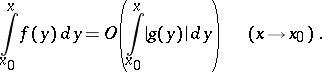Formulas containing the symbols and 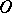are read only from the left to the right; however, this does not exclude that certain formulas remain true when read from the right to the left. The symbols and 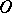for functions of a complex variable and for functions of several variables are introduced in the same way as it was done above for functions of one real variable.

Comments

The symbols and ( "little oh symbollittle oh" and "big Oh symbolbig Oh" ) were introduced by E. Landau.

References

[a1]	G.H. Hardy, "A course of pure mathematics" , Cambridge Univ. Press (1975)
[a2]	E. Landau, "Grundlagen der Analysis" , Akad. Verlagsgesellschaft (1930)