Bicharacteristic
bicharacteristic strip, of a linear partial differential operator
A line of tangency of any two characteristics (cf. Characteristic)
 |
of this linear partial differential operator. If the parameter  is introduced on the bicharacteristic strip, then its equations
is introduced on the bicharacteristic strip, then its equations 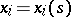 ,
,  , are defined by solving a system of
, are defined by solving a system of 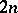 ordinary differential equations
ordinary differential equations
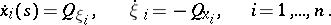 | (*) |
Here  is the principal symbol of the linear partial differential operator, the dot indicates differentiation with respect to the parameter
is the principal symbol of the linear partial differential operator, the dot indicates differentiation with respect to the parameter  and, if
and, if 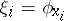 , the equation
, the equation 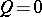 is the characteristic equation of the differential operator. Thus, the solution
is the characteristic equation of the differential operator. Thus, the solution 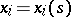 ,
, 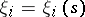 ,
, 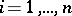 , of the system (*) for
, of the system (*) for 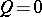 defines the bicharacteristic strip
defines the bicharacteristic strip 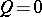 . This bicharacteristic strip belongs to the characteristic
. This bicharacteristic strip belongs to the characteristic 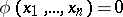 , i.e.
, i.e. 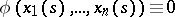 , if the equations
, if the equations
 |
and
 |
are valid for at least one value of  , then it follows that they are valid for all values of
, then it follows that they are valid for all values of  .
.
References
| [1] | R. Courant, D. Hilbert, "Methods of mathematical physics. Partial differential equations" , 2 , Interscience (1965) (Translated from German) |
Comments
The projections 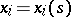 ,
,  , into
, into  -space are called the bicharacteristic curves (or rays). The bicharacteristic curves are tangent to the characteristic hypersurfaces
-space are called the bicharacteristic curves (or rays). The bicharacteristic curves are tangent to the characteristic hypersurfaces 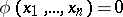 due to the homogeneity of the principal symbol, as a function of
due to the homogeneity of the principal symbol, as a function of 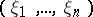 , of degree equal to the order of the linear partial differential operator (cf. also Principal part of a differential operator; Symbol of an operator).
, of degree equal to the order of the linear partial differential operator (cf. also Principal part of a differential operator; Symbol of an operator).
Nowadays, the standard reference on these matters is [a1], or the older, more concise, [a2].
References
| [a1] | L.V. Hörmander, "The analysis of linear partial differential operators" , 1 , Springer (1983) pp. 271; 302 |
| [a2] | L. Hörmander, "Linear partial differential operators" , Springer (1963) pp. 29; 31 |
Bicharacteristic. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bicharacteristic&oldid=15970