Bernoulli equation
From Encyclopedia of Mathematics
An ordinary first-order differential equation
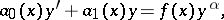 |
where 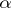 is a real number other than zero or one. This equation was first studied by J. Bernoulli [1]. The substitution
is a real number other than zero or one. This equation was first studied by J. Bernoulli [1]. The substitution 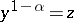 converts the Bernoulli equation to a linear inhomogeneous first-order equation, [2]. If
converts the Bernoulli equation to a linear inhomogeneous first-order equation, [2]. If 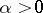 , the solution of the Bernoulli equation is
, the solution of the Bernoulli equation is 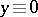 ; if
; if 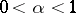 , at some points the solution is no longer single-valued. Equations of the type
, at some points the solution is no longer single-valued. Equations of the type
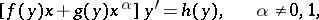 |
are also Bernoulli equations if 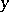 is considered as the independent variable, while
is considered as the independent variable, while  is an unknown function of
is an unknown function of 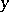 .
.
References
| [1] | J. Bernoulli, Acta Erud. (1695) pp. 59–67; 537–557 |
| [2] | E. Kamke, "Differentialgleichungen: Lösungen und Lösungsmethoden" , 1. Gewöhnliche Differentialgleichungen , Chelsea, reprint (1971) |
Comments
References
| [a1] | E.L. Ince, "Ordinary differential equations" , Dover, reprint (1956) |
How to Cite This Entry:
Bernoulli equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bernoulli_equation&oldid=15844
Bernoulli equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bernoulli_equation&oldid=15844
This article was adapted from an original article by N.Kh. Rozov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article