Fourier hyperfunction
The theory of Fourier hyperfunctions is a variant or generalization of the theory of Fourier transforms to wider classes of (generalized) functions than the usual ones (cf. also Fourier transform). The keyword of this theory is infra-exponential growth, that is, growth less than any exponential type. Fourier transforms of functions of infra-exponential growth were considered by L. Carleman. A general theory of Fourier hyperfunctions was proposed by M. Sato at the same time as his theory of hyperfunctions, in which the transformed objects can be interpreted naturally (cf. also Hyperfunction).
Sato gave a justification for the one-variable case in [a1]. A foundation for the general  -dimensional case was given by T. Kawai [a2], with an application to the theory of linear partial differential equations with constant coefficients. Since then, various extensions of the theory have been proposed.
-dimensional case was given by T. Kawai [a2], with an application to the theory of linear partial differential equations with constant coefficients. Since then, various extensions of the theory have been proposed.
As is usual in Fourier theory, generalization can proceed along three lines: as the dual, as the generalized limit, or as the derivative of the classical Fourier transform.
Duality theory.
Let  be the fundamental space of test functions
be the fundamental space of test functions 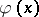 that can be analytically continued to a strip
that can be analytically continued to a strip  and that satisfy in this strip the estimate
and that satisfy in this strip the estimate
 | (a1) |
This is the inductive limit of the space 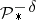 defined by a fixed
defined by a fixed 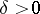 as above, endowed with the norm
as above, endowed with the norm
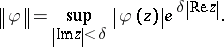 |
The same limit space is obtained if 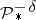 is replaced by the Hilbert space of holomorphic functions such that
is replaced by the Hilbert space of holomorphic functions such that 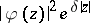 is integrable on
is integrable on 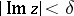 . Hence,
. Hence,  becomes a well-behaved space of type (DFS) (cf. also Generalized functions, space of), even nuclear (cf. also Nuclear space), and one can consider the dual space
becomes a well-behaved space of type (DFS) (cf. also Generalized functions, space of), even nuclear (cf. also Nuclear space), and one can consider the dual space 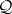 of
of 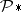 , which is called the space of Fourier hyperfunctions.
, which is called the space of Fourier hyperfunctions.  is of type FS and nuclear. The classical Fourier transform is easily seen to not only preserve the space
is of type FS and nuclear. The classical Fourier transform is easily seen to not only preserve the space 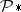 , but also to act on it as a topological isomorphism. Thus, one can define the Fourier transform on
, but also to act on it as a topological isomorphism. Thus, one can define the Fourier transform on 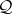 by duality; it also gives an isomorphism. Since there is a continuous injection with dense range
by duality; it also gives an isomorphism. Since there is a continuous injection with dense range 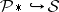 , the same is true for
, the same is true for 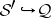 . In this way a generalization of the Fourier transform is obtained that is wider than the Schwartz theory of tempered distributions.
. In this way a generalization of the Fourier transform is obtained that is wider than the Schwartz theory of tempered distributions.
Boundary value representation.
Fourier hyperfunctions can be obtained as ideal limits of holomorphic functions with a certain growth restriction. Similarly to the boundary value representation of ordinary hyperfunctions by defining holomorphic functions (cf. also Hyperfunction), a Fourier hyperfunction admits the following representation:
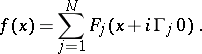 | (a2) |
Here, each 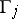 is a convex open cone with vertex at the origin and
is a convex open cone with vertex at the origin and 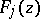 is holomorphic on the wedge
is holomorphic on the wedge 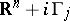 satisfying the infra-exponential estimate "for all e>0, Fjz= OeeRez" locally uniformly in
satisfying the infra-exponential estimate "for all e>0, Fjz= OeeRez" locally uniformly in  , where
, where 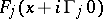 denotes its abstract limit to the real axis. The duality with
denotes its abstract limit to the real axis. The duality with 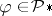 is represented by the integral
is represented by the integral
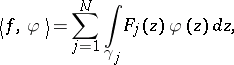 |
where  is a path in the intersection of
is a path in the intersection of 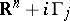 with the domain of definition of
with the domain of definition of 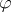 . The value of the integral does not depend on the choice of
. The value of the integral does not depend on the choice of 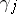 . For the validity of all these it suffices that each
. For the validity of all these it suffices that each 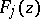 is defined only on the part of the corresponding wedge lying in a strip neighbourhood
is defined only on the part of the corresponding wedge lying in a strip neighbourhood 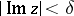 of the real axis where
of the real axis where 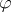 is defined.
is defined.
The kernel function 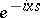 of the Fourier transform is not a test function itself, but if
of the Fourier transform is not a test function itself, but if 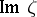 is restricted to some convex open cone
is restricted to some convex open cone 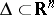 , then
, then  is exponentially decreasing in
is exponentially decreasing in 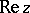 on
on 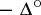 , where
, where
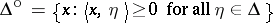 |
denotes the dual cone of 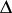 . Thus, if each
. Thus, if each  is exponentially decreasing when
is exponentially decreasing when 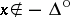 , then
, then 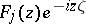 is exponentially decreasing everywhere in
is exponentially decreasing everywhere in 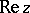 when
when  is in
is in  and small enough. Thus, the Fourier transform can be calculated as the abstract limit
and small enough. Thus, the Fourier transform can be calculated as the abstract limit 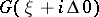 of the function
of the function
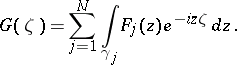 | (a3) |
For the general case one uses a partition of unity 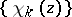 such that each
such that each 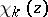 is exponentially decreasing when
is exponentially decreasing when 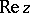 is outside a convex cone
is outside a convex cone 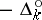 , and one sets
, and one sets
 |
where each 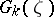 is calculated by (a3) with
is calculated by (a3) with 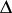 replaced by
replaced by  and
and 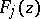 by
by 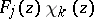 . If the partition is made of orthants
. If the partition is made of orthants  with
with 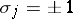 , then one can take as the partition function
, then one can take as the partition function  .
.
Localization.
There are many possibilities to extend the Fourier transform by means of duality, based on various fundamental spaces of test functions stable under the Fourier transform. If one chooses a fundamental space smaller than 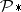 , one obtain a wider extension thereof. The most significant feature of Fourier hyperfunctions among such is localizability. Namely, one can define a sheaf
, one obtain a wider extension thereof. The most significant feature of Fourier hyperfunctions among such is localizability. Namely, one can define a sheaf 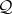 on the directional compactification
on the directional compactification 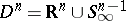 such that the above-introduced space of Fourier hyperfunctions agrees with the global section space
such that the above-introduced space of Fourier hyperfunctions agrees with the global section space 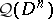 . In this sense, infra-exponential growth is the best possible choice. The sheaf
. In this sense, infra-exponential growth is the best possible choice. The sheaf 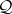 of Fourier hyperfunctions is constructed from the sheaf
of Fourier hyperfunctions is constructed from the sheaf 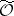 of germs of holomorphic functions with infra-exponential growth in the real direction as its
of germs of holomorphic functions with infra-exponential growth in the real direction as its  th derived sheaf:
th derived sheaf: 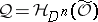 . The sheaf
. The sheaf 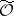 is considered as living on
is considered as living on 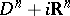 , the growth condition describing the stalks at the points at infinity. Thus, when restricted to the finite points,
, the growth condition describing the stalks at the points at infinity. Thus, when restricted to the finite points, 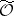 reduces to
reduces to 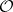 and
and  to the sheaf
to the sheaf 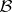 of usual hyperfunctions. Thanks to fundamental cohomology vanishing theorems for the sheaf
of usual hyperfunctions. Thanks to fundamental cohomology vanishing theorems for the sheaf 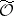 similar to those for
similar to those for  , the space of Fourier hyperfunctions on an open set
, the space of Fourier hyperfunctions on an open set 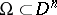 can be represented by the global cohomology group
can be represented by the global cohomology group  , and this in turn can be represented by the covering cohomology: Choosing
, and this in turn can be represented by the covering cohomology: Choosing 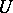 to be
to be 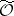 -Stein, i.e. cohomologically trivial for
-Stein, i.e. cohomologically trivial for 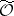 (cf. also Stein manifold), one obtains, e.g.,
(cf. also Stein manifold), one obtains, e.g.,
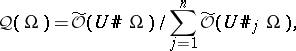 |
where
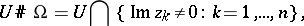 |
 |
This can be interpreted as the local boundary value representation
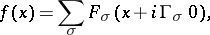 |
where
 |
A more sophisticated choice of an 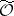 -Stein covering of
-Stein covering of 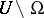 justifies a local boundary value representation of the form (a2) which is valid on
justifies a local boundary value representation of the form (a2) which is valid on  , just as in the case of ordinary hyperfunctions.
, just as in the case of ordinary hyperfunctions.
The sheaf 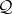 can be constructed also via duality, as in Martineau's theory for ordinary hyperfunctions: For each compact subset
can be constructed also via duality, as in Martineau's theory for ordinary hyperfunctions: For each compact subset  , one denotes by
, one denotes by 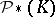 the space of
the space of  such that there is a neighbourhood
such that there is a neighbourhood 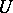 of
of 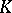 in
in 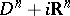 such that
such that 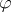 is holomorphic in
is holomorphic in  and is exponentially decreasing at infinity.
and is exponentially decreasing at infinity.
Notice that the decay condition is meaningful only at points at infinity of 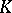 . Then its dual
. Then its dual  gives the space of Fourier hyperfunctions
gives the space of Fourier hyperfunctions  supported by
supported by 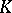 . General sections of Fourier hyperfunctions can be represented as obvious equivalence classes of locally finite sums of these.
. General sections of Fourier hyperfunctions can be represented as obvious equivalence classes of locally finite sums of these.  can be expressed by the relative cohomology group
can be expressed by the relative cohomology group 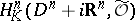 . This is an extension of Martineau–Harvey duality in the theory of ordinary hyperfunctions. The case
. This is an extension of Martineau–Harvey duality in the theory of ordinary hyperfunctions. The case  corresponds to that for global Fourier hyperfunctions, given at the beginning.
corresponds to that for global Fourier hyperfunctions, given at the beginning.
Contrary to the general feeling, Schwartz tempered distributions can be localized in a similar way: One can consider a sheaf 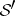 on
on  of tempered distributions defined via duality in the same way as above. The notion of localization of
of tempered distributions defined via duality in the same way as above. The notion of localization of 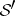 with respect to the directional coordinates is useful. The global sections of
with respect to the directional coordinates is useful. The global sections of  on
on 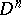 give the usual space of tempered distributions, whereas its global sections on
give the usual space of tempered distributions, whereas its global sections on 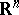 lead to the usual space
lead to the usual space 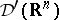 of distributions (cf. also Nuclear space). In this case the importance of the compactification
of distributions (cf. also Nuclear space). In this case the importance of the compactification 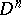 is not clear, because
is not clear, because 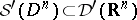 , hence information on
, hence information on 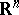 suffices to determine a tempered distribution, as is usually done.
suffices to determine a tempered distribution, as is usually done.
In the case of Fourier hyperfunctions, however, this relation is more complicated: There is a canonical surjection 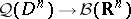 . The surjectivity is a part of the flabbiness of the sheaf
. The surjectivity is a part of the flabbiness of the sheaf 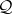 , which can be established analogously to the case of
, which can be established analogously to the case of  (cf. also Flabby sheaf). As usual, the extension is not unique. Especially, there are Fourier hyperfunctions supported at the points at infinity. The concrete Morimoto–Yoshino example of a Fourier hyperfunction with one point support at
(cf. also Flabby sheaf). As usual, the extension is not unique. Especially, there are Fourier hyperfunctions supported at the points at infinity. The concrete Morimoto–Yoshino example of a Fourier hyperfunction with one point support at 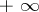 in one variable is given as the boundary value
in one variable is given as the boundary value  of the function defined by the integral
of the function defined by the integral
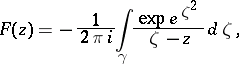 |
where 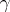 is a simple path starting and ending at
is a simple path starting and ending at 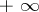 and passing through the region where
and passing through the region where 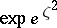 is decreasing, say
is decreasing, say  .
.
As a consequence of localizability, one can consider the support of Fourier hyperfunctions. Furthermore, by the flabbiness of the sheaf 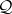 , one can decompose the support of a given Fourier hyperfunction according to any covering by closed subsets of
, one can decompose the support of a given Fourier hyperfunction according to any covering by closed subsets of 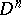 . In particular, given a decomposition of
. In particular, given a decomposition of 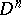 by closed convex cones
by closed convex cones 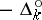 with vertex at the origin, or, more generally, by closed subsets which are asymptotically such, one can decompose
with vertex at the origin, or, more generally, by closed subsets which are asymptotically such, one can decompose 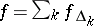 accordingly, in such a way that
accordingly, in such a way that  . Then the Fourier transform of each can be calculated as the inner product
. Then the Fourier transform of each can be calculated as the inner product
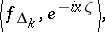 |
which is meaningful for 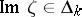 , where
, where 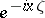 can serve as a test function in
can serve as a test function in  . Thus, the Fourier transform of
. Thus, the Fourier transform of 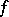 can be calculated as the sum of the boundary values of these from respective wedges. In practical calculations one does not have to replace the defining functions to realize
can be calculated as the sum of the boundary values of these from respective wedges. In practical calculations one does not have to replace the defining functions to realize  . For example, the Fourier transform of the Poisson distribution
. For example, the Fourier transform of the Poisson distribution  can be calculated by means of its natural defining function
can be calculated by means of its natural defining function 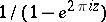 by a suitable choice of the integral path corresponding to the decomposition of the support to
by a suitable choice of the integral path corresponding to the decomposition of the support to 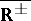 , giving
, giving  .
.
The following generalization of Paley–Wiener type holds: Let 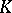 be a convex compact subset of
be a convex compact subset of 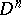 . (Here, "convex" means that
. (Here, "convex" means that 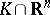 is convex in the usual sense and that
is convex in the usual sense and that  generates a convex cone
generates a convex cone 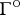 , called the asymptotic cone of
, called the asymptotic cone of 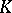 .) Then
.) Then 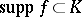 if and only if there exists a
if and only if there exists a 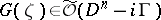 such that for any
such that for any 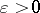 and
and 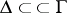 ,
, 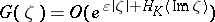 uniformly on
uniformly on 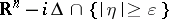 , and such that
, and such that 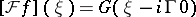 .
.
Microlocalization.
Just as in the case of ordinary hyperfunctions, one can consider microlocal regularity for Fourier hyperfunctions: 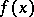 is said to be micro-analytic at
is said to be micro-analytic at 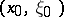 if it admits a local boundary value representation (a2) that is valid in a neighbourhood of
if it admits a local boundary value representation (a2) that is valid in a neighbourhood of 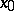 such that the half-space
such that the half-space  meets all of
meets all of 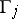 . This is equivalent to saying that in a neighbourhood of
. This is equivalent to saying that in a neighbourhood of 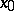 ,
, 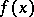 can be written as the sum
can be written as the sum 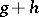 , where
, where  comes from a local section of
comes from a local section of  and
and 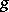 is a global Fourier hyperfunction whose Fourier transform is zero (exponentially decreasing) on a conic neighbourhood of the direction
is a global Fourier hyperfunction whose Fourier transform is zero (exponentially decreasing) on a conic neighbourhood of the direction 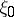 . The set of points where
. The set of points where  is not micro-analytic is called the singular spectrum or the analytic wavefront set of
is not micro-analytic is called the singular spectrum or the analytic wavefront set of  , and is denoted by
, and is denoted by 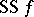 or
or 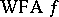 . This notion includes not only that of directional analyticity, but also the directional growth property of
. This notion includes not only that of directional analyticity, but also the directional growth property of 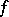 . For example, if
. For example, if 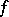 is analytic on a strip neighbourhood of the real axis but not of infra-exponential growth, then
is analytic on a strip neighbourhood of the real axis but not of infra-exponential growth, then 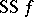 may contain
may contain 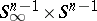 .
.
One can introduce the sheaf of Fourier microfunctions representing the microlocal singularities of the Fourier hyperfunctions. This sheaf is flabby, and consequently one can decompose the singular spectrum of Fourier hyperfunctions according to any closed covering [a6].
This notion may be effectively employed for certain problems in global analysis on unbounded domains [a5].
Relation to other (generalized) functions.
In addition to the space of tempered distributions 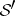 , the space of Fourier hyperfunctions contains the space of ultra-distributions of Gevrey index
, the space of Fourier hyperfunctions contains the space of ultra-distributions of Gevrey index  and of growth order
and of growth order  as a subspace invariant under the Fourier transform. Hyperfunctions with compact supports can be canonically considered as Fourier hyperfunctions. General hyperfunctions can be considered as Fourier hyperfunctions after extension to
as a subspace invariant under the Fourier transform. Hyperfunctions with compact supports can be canonically considered as Fourier hyperfunctions. General hyperfunctions can be considered as Fourier hyperfunctions after extension to 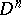 , but the extension is not unique and the ambiguity of extension influences the result of the Fourier transform in an essential manner. A measurable function of infra-exponential growth (in the sense of the essential supremum) can be canonically considered as a Fourier hyperfunction. Conversely, any Fourier hyperfunction can be represented as the derivative of such a function by a local operator
, but the extension is not unique and the ambiguity of extension influences the result of the Fourier transform in an essential manner. A measurable function of infra-exponential growth (in the sense of the essential supremum) can be canonically considered as a Fourier hyperfunction. Conversely, any Fourier hyperfunction can be represented as the derivative of such a function by a local operator 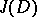 , that is, an infinite-order differential operator whose symbol is an entire function of order
, that is, an infinite-order differential operator whose symbol is an entire function of order  and of minimal type.
and of minimal type.
Extensions.
Modified Fourier hyperfunctions.
There are many choices for the compactification of 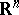 or
or 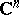 , and one can consider corresponding versions of Fourier hyperfunctions. The most important one is defined on the real axis in the full directional compactification
, and one can consider corresponding versions of Fourier hyperfunctions. The most important one is defined on the real axis in the full directional compactification 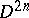 of
of 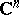 , and is called the space of modified Fourier hyperfunctions. While the typical shape of a complex fundamental neighbourhood of a real point at infinity
, and is called the space of modified Fourier hyperfunctions. While the typical shape of a complex fundamental neighbourhood of a real point at infinity 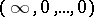 in the space
in the space 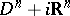 of standard Fourier hyperfunctions has the form
of standard Fourier hyperfunctions has the form
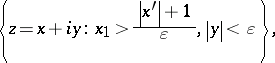 |
the shape in  for a modified Fourier hyperfunction is
for a modified Fourier hyperfunction is
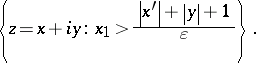 |
The sheaf  of holomorphic functions of infra-exponential growth for this modified topology is defined in an obvious manner. The sheaf
of holomorphic functions of infra-exponential growth for this modified topology is defined in an obvious manner. The sheaf  of modified Fourier hyperfunctions is defined from the former by the same procedure as in the standard situation. The space
of modified Fourier hyperfunctions is defined from the former by the same procedure as in the standard situation. The space 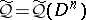 of global modified Fourier hyperfunctions is the dual of the space
of global modified Fourier hyperfunctions is the dual of the space 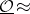 of exponentially decreasing holomorphic functions defined on a "conical" complex neighbourhood
of exponentially decreasing holomorphic functions defined on a "conical" complex neighbourhood 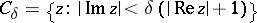 of the real axis. This modified version can be used to distinguish the analytic singular support of (Fourier) hyperfunctions: Let
of the real axis. This modified version can be used to distinguish the analytic singular support of (Fourier) hyperfunctions: Let 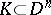 be a convex compact subset with asymptotic cone
be a convex compact subset with asymptotic cone 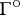 . Then
. Then 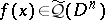 is a section of
is a section of 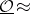 outside
outside  if and only if there is a representation
if and only if there is a representation 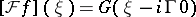 by
by  as follows: For any
as follows: For any 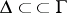 and for any
and for any 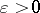 , one can find a
, one can find a 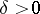 such that
such that 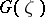 is holomorphic in
is holomorphic in  and
and 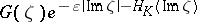 is of infra-exponential growth in
is of infra-exponential growth in 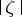 locally uniformly as
locally uniformly as 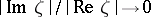 . This generalizes a similar result of L. Ehrenpreis for usual
. This generalizes a similar result of L. Ehrenpreis for usual 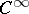 singular supports.
singular supports.
The profitability of the idea of a modified Fourier hyperfunction was discovered by M. Sato and T. Kawai in their joint researches (see [a2]). Its foundation was developed in [a4] in detail. Further generalizations have made by several people (see e.g. [a8], [a9]).
Fourier ultra-hyperfunctions.
Functions of exponential growth cannot be canonically considered as Fourier hyperfunctions. The theory of Fourier ultra-hyperfunctions enables one to treat them naturally: The fundamental space of test functions in this theory is defined on a neighbourhood of a convex tube of base 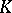 , and has decay of
, and has decay of 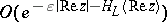 for some
for some 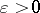 , where
, where 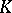 and
and 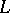 are two convex compact sets. The Fourier transform maps this space isomorphically onto a similar space, with
are two convex compact sets. The Fourier transform maps this space isomorphically onto a similar space, with 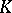 and
and 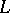 replaced by
replaced by 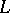 and
and  . The elements of the dual space of this space are called Fourier ultra-hyperfunctions. They can also be given via the relative cohomology group of the corresponding sheaf of holomorphic functions with suitable growth. Thus, in short, the growth of the defining functions is allowed to be of a fixed exponential type, but as compensation for that, its "supports" as analytic functionals bulk to a tube, and no local theory is available [a3]. This theory is useful for identifying special kinds of entire functions of exponential type.
. The elements of the dual space of this space are called Fourier ultra-hyperfunctions. They can also be given via the relative cohomology group of the corresponding sheaf of holomorphic functions with suitable growth. Thus, in short, the growth of the defining functions is allowed to be of a fixed exponential type, but as compensation for that, its "supports" as analytic functionals bulk to a tube, and no local theory is available [a3]. This theory is useful for identifying special kinds of entire functions of exponential type.
Fourier hyperfunctions on manifolds.
On a real-analytic open manifold 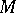 one can introduce the sheaf of Fourier hyperfunctions, extending the usual sheaf of hyperfunctions, whose base is the compactification of
one can introduce the sheaf of Fourier hyperfunctions, extending the usual sheaf of hyperfunctions, whose base is the compactification of 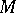 . The suitable growth condition, which is not necessarily infra-exponential, is determined from the boundary geometry of
. The suitable growth condition, which is not necessarily infra-exponential, is determined from the boundary geometry of  . This is effectively used to study the spectral properties of elliptic operators or the boundary behaviour of the manifold itself [a10], [a11].
. This is effectively used to study the spectral properties of elliptic operators or the boundary behaviour of the manifold itself [a10], [a11].
Analogues for other types of integral transforms.
Similar ideas can be employed to generalize the Mellin transform, the Radon transform and other integral transforms (see, e.g., [a12], [a13], [a14], [a15]).
References
| [a1] | M. Sato, "Theory of hyperfunctions" SÛgaku , 10 (1958) pp. 1–27 (In Japanese) |
| [a2] | T. Kawai, "On the theory of Fourier hyperfunctions and its applications to partial differential equations with constant coefficients" J. Fac. Sci. Univ. Tokyo Sec. 1A , 17 (1970) pp. 467–517 |
| [a3] | Y.S. Park, M. Morimoto, "Fourier ultra hyperfunctions in the Euclidean  -space" J. Fac. Sci. Univ. Tokyo Sec. 1A , 20 (1973) pp. 121–127 -space" J. Fac. Sci. Univ. Tokyo Sec. 1A , 20 (1973) pp. 121–127 |
| [a4] | Y. Saburi, "Fundamental properties of modified Fourier hyperfunctions" Tokyo J. Math. , 4 (1985) pp. 231–273 |
| [a5] | A. Kaneko, "On the global existence of real analytic solutions of linear partial differential equations on unbounded domain" J. Fac. Sci. Univ. Tokyo Sec. 1A , 32 (1985) pp. 319–372 |
| [a6] | A. Kaneko, "On the flabbiness of the sheaf of Fourier hyperfunctions" Sci. Pap. Coll. Gen. Educ. Univ. Tokyo , 36 (1986) pp. 1–14 |
| [a7] | A. Kaneko, "Introduction to hyperfunctions" , Kluwer Acad. Publ. (1988) |
| [a8] | S. Nagamachi, "The theory of vector valued Fourier hyperfunctions of mixed type I" Publ. RIMS Kyoto Univ. , 17 (1981) pp. 25–63 |
| [a9] | Y. Ito, "Fourier hyperfunctions of general type" J. Math. Kyoto Univ. , 38 (1988) pp. 213–265 |
| [a10] | M. Morimoto, "Analytic functionals on the Lie sphere" Tokyo J. Math. , 3 (1980) pp. 1–35 |
| [a11] | T. Oshima, Y. Saburi, M. Wakayama, "Paley Wiener theorems on a symmetric space and its applications" Diff. Geom. Appl. , 1 (1991) pp. 247–278 |
| [a12] | H. Komatsu, "Multipliers for Laplace hyperfunctions: A justification of Heaviside rules" Proc. Steklov Inst. Math. , 203 (1994) pp. 271–279 |
| [a13] | T. Takiguchi, A. Kaneko, "Radon transform of hyperfunctions and support theorem" Hokkaido Math. J. , 24 (1995) pp. 63–103 |
| [a14] | B. Ziemian, "The Mellin transformation and multidimensional generalized Taylor expansions of singular functions" J. Fac. Sci. Univ. Tokyo Sec. 1A , 36 (1989) pp. 263–295 |
| [a15] | Z. Szmydt, B. Ziemian, "Laplace distributions and hyperfunctions on  " J. Math. Sci. Univ. Tokyo , 5 (1998) pp. 41–74 " J. Math. Sci. Univ. Tokyo , 5 (1998) pp. 41–74 |
Fourier hyperfunction. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Fourier_hyperfunction&oldid=15571