Power residue

modulo

An integer for which the congruence

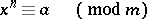is solvable for a given integer 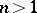. The number is called a residue of degree modulo 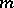. If this congruence is not solvable, then is called a non-residue of degree modulo . When , the power residues and non-residues are said to be quadratic, when , cubic, and when 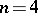, biquadratic.

In the case of a prime modulus 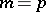, the question of the solvability of the congruence 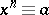(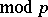) can be answered by using Euler's test: If 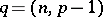, then for the congruence 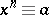(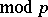) to be solvable it is necessary and sufficient that

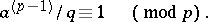When this condition is fulfilled, the original congruence has 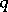different solutions modulo 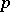. It follows from this test that among the numbers 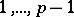there are exactly 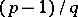residues and 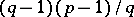non-residues of degree modulo 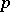. See Distribution of power residues and non-residues.

Comments

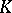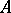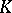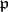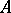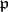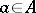As in the case of quadratic residues one defines a power-residue symbol. Let be a number field containing the -th roots of unity. Let be the ring of integers of and let be a prime ideal of . Let be relatively prime to and . If 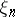is a primitive -th root of unity, one has

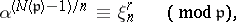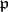where is the norm of , i.e. the number of elements of 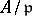. One now defines the power-residue symbol

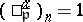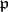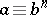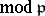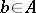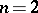If , then is an -th power residue modulo , i.e. () is solvable for . If , and 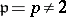, one finds back the quadratic-residue symbol, cf. Legendre symbol.

There also exist power-residue reciprocity laws, cf. e.g. [a2], which specialize to the quadratic reciprocity law if , .

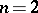References

[a1]	W. Narkiewicz, "Elementary and analytic theory of algebraic numbers" , Springer & PWN (1990) pp. 394ff
[a2]	J. Neukirch, "Class field theory" , Springer (1986) pp. Chapt. IV, §9