Projective normal
A generalization of the concept of a normal in metric geometry. Distinct from the latter, where a normal is totally determined by the tangent plane to a surface (i.e. the first-order neighbourhood), this does not hold in projective geometry. Even terms of the first order of smallness do not determine the vertices of a coordinate tetrahedron not lying in the tangent plane (i.e. for a chosen Darboux quadric it is impossible to construct a single self-polar tetrahedron). This is a natural situation: the projective group is much larger than the group of motions, and therefore its invariants must be of higher order; but even in the fourth-order neighbourhood there is no unique straight line that could be taken as the third axis of a tetrahedron. In this way one obtains, e.g.:
the Wilczynski directrix
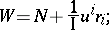 |
the Green edge
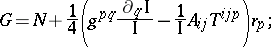 |
the Čech axis
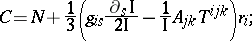 |
and the Fubini normal
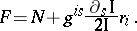 |
Here 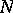 is the affine normal.
is the affine normal.
They all lie in one plane.
References
| [1] | P.A. Shirokov, A.P. Shirokov, "Differentialgeometrie" , Teubner (1962) (Translated from Russian) |
| [2] | A.P. Norden, "Spaces with an affine connection" , Nauka , Moscow-Leningrad (1976) (In Russian) |
| [3] | S.P. Finikov, "Projective differential geometry" , Moscow-Leningrad (1937) (In Russian) |
Comments
References
| [a1] | G. Bol, "Projektive differentialgeometrie" , I, II , Vandenhoeck & Ruprecht (1950) |
| [a2] | E. Cartan, "Leçons sur la théorie des espaces à connexion projective" , Gauthier-Villars (1937) |
| [a3] | G. Fubini, E. Čech, "Introduction á la géométrie projective différentielle des surfaces" , Gauthier-Villars (1931) |
Projective normal. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Projective_normal&oldid=15386