Riemann surfaces, classification of
Studies of Riemann surfaces related to the behaviour of different classes of functions on these surfaces.
A complex-valued function 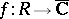 on a Riemann surface
on a Riemann surface  is said to be analytic on
is said to be analytic on 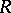 if for any point
if for any point 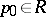 there exists a neighbourhood
there exists a neighbourhood  and a local uniformizing parameter
and a local uniformizing parameter 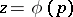 ,
,  , mapping
, mapping 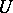 homeomorphically onto the unit disc
homeomorphically onto the unit disc  , such that the composite function
, such that the composite function 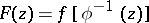 is a single-valued analytic function on
is a single-valued analytic function on 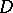 . Similarly, one can define on Riemann surfaces real-valued and complex-valued harmonic functions, subharmonic functions, etc. Let
. Similarly, one can define on Riemann surfaces real-valued and complex-valued harmonic functions, subharmonic functions, etc. Let  be some conformally-invariant class of functions on the Riemann surface
be some conformally-invariant class of functions on the Riemann surface  containing the constants. The problem of the classification of Riemann surfaces in its simplest formulation consists in determining conditions under which a given Riemann surface
containing the constants. The problem of the classification of Riemann surfaces in its simplest formulation consists in determining conditions under which a given Riemann surface 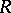 will or will not belong to the class
will or will not belong to the class 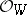 of those Riemann surfaces for which the class
of those Riemann surfaces for which the class 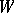 on them consists only of the constants. The classification theory of Riemann surfaces arose in the 20th century from the classical Riemann theorem on the conformal mapping of simply-connected Riemann surfaces, the problem of types, the problem of the existence of a Green function of a Riemann surface, and the concept of the ideal boundary of a Riemann surface.
on them consists only of the constants. The classification theory of Riemann surfaces arose in the 20th century from the classical Riemann theorem on the conformal mapping of simply-connected Riemann surfaces, the problem of types, the problem of the existence of a Green function of a Riemann surface, and the concept of the ideal boundary of a Riemann surface.
Riemann's mapping theorem states that any simply-connected Riemann surface 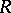 can be mapped conformally (and, hence, homeomorphically) onto a domain
can be mapped conformally (and, hence, homeomorphically) onto a domain 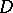 of exactly one of the following types:
of exactly one of the following types: 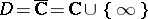 — the extended complex plane (the case of a Riemann surface
— the extended complex plane (the case of a Riemann surface 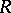 of elliptic type);
of elliptic type); 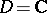 — the finite complex plane (
— the finite complex plane (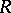 is of parabolic type); or
is of parabolic type); or 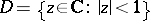 — the unit disc (
— the unit disc (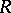 is of hyperbolic type). Since the elliptic case differs from the others already from the topological point of view, the difficult problem of recognizing whether a given Riemann surface
is of hyperbolic type). Since the elliptic case differs from the others already from the topological point of view, the difficult problem of recognizing whether a given Riemann surface 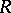 is of hyperbolic or parabolic type is still left. This is the classical problem of types, which is unsolved until now (1991). It is known that a closed Riemann surface of genus
is of hyperbolic or parabolic type is still left. This is the classical problem of types, which is unsolved until now (1991). It is known that a closed Riemann surface of genus 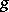 for
for 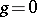 is of elliptic type, for
is of elliptic type, for 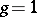 it is of parabolic type, and for
it is of parabolic type, and for 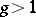 of hyperbolic type; therefore, the problem of types is mainly important for open Riemann surfaces. In the case of an arbitrary, not necessarily simply-connected, Riemann surface
of hyperbolic type; therefore, the problem of types is mainly important for open Riemann surfaces. In the case of an arbitrary, not necessarily simply-connected, Riemann surface  , its type is the same as the type of its universal covering surface (see Universal covering)
, its type is the same as the type of its universal covering surface (see Universal covering)  , which is always simply connected.
, which is always simply connected.
For simply-connected finite Riemann surfaces 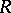 , the problem of finding a conformal mapping from
, the problem of finding a conformal mapping from 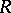 onto the unit disc
onto the unit disc  is equivalent to the problem of finding the Green function
is equivalent to the problem of finding the Green function 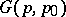 for
for 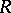 , i.e. a positive harmonic function with logarithmic singularity of the form
, i.e. a positive harmonic function with logarithmic singularity of the form 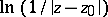 at the pole
at the pole 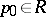 (
(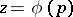 is a parameter in a neighbourhood of
is a parameter in a neighbourhood of 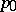 ,
, 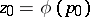 ), vanishing at all points of the boundary
), vanishing at all points of the boundary 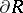 . The Green function can be also constructed for multiply-connected finite Riemann surfaces of hyperbolic type. In the case of an arbitrary open Riemann surface
. The Green function can be also constructed for multiply-connected finite Riemann surfaces of hyperbolic type. In the case of an arbitrary open Riemann surface  one can construct an exhaustion
one can construct an exhaustion 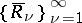 of the surface
of the surface 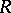 by finite Riemann surfaces
by finite Riemann surfaces 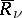 with boundary and having Green functions
with boundary and having Green functions
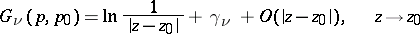 |
(or 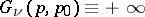 from some index
from some index  onwards), and such that
onwards), and such that 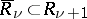 ,
,  . The constant
. The constant 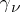 ,
,  , is called the Robin constant of the Riemann surface
, is called the Robin constant of the Riemann surface 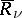 ;
; 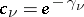 is the capacity of the boundary
is the capacity of the boundary 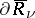 (relative to the fixed pole
(relative to the fixed pole 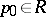 ). When
). When  tends to
tends to 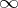 , the values of
, the values of 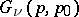 and
and 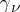 can only increase. The Green function of an open Riemann surface
can only increase. The Green function of an open Riemann surface  is defined as the limit
is defined as the limit 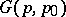 of the increasing sequence
of the increasing sequence 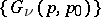 if such a limit exists; otherwise, if
if such a limit exists; otherwise, if
 |
one says that the Riemann surface  does not have a Green function. The existence or non-existence of the Green function is independent of the choice of the pole
does not have a Green function. The existence or non-existence of the Green function is independent of the choice of the pole  . The class of Riemann surfaces for which the Green function does not exist is denoted by
. The class of Riemann surfaces for which the Green function does not exist is denoted by 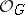 . In other words, the class
. In other words, the class  is characterized by
is characterized by
 |
moreover, these relations are independent of the choice of the pole as well.
Let 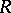 be an open Riemann surface and let
be an open Riemann surface and let 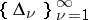 be a so-called defining sequence of closed domains
be a so-called defining sequence of closed domains 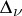 on
on  , i.e. a sequence such that: 1) the boundary of
, i.e. a sequence such that: 1) the boundary of 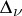 is a simple closed curve on
is a simple closed curve on 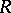 ; 2)
; 2) 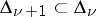 ,
,  ; 3)
; 3) 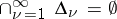 , i.e. the
, i.e. the 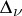 are not compact in
are not compact in 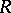 . Two defining sequences
. Two defining sequences 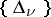 and
and 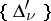 are equivalent if to each
are equivalent if to each  there correspond
there correspond  and
and 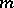 such that
such that 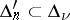 and
and 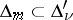 . Equivalence classes of defining sequences are called boundary elements of
. Equivalence classes of defining sequences are called boundary elements of 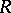 , and the set of all boundary elements forms the ideal boundary
, and the set of all boundary elements forms the ideal boundary 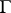 of
of  , considered as a topological space. For instance, the ideal boundary of the unit disc
, considered as a topological space. For instance, the ideal boundary of the unit disc 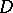 consists of one boundary element. Note that the Green function of an open Riemann surface
consists of one boundary element. Note that the Green function of an open Riemann surface 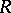 , unlike the case of a hyperbolic finite Riemann surface, does not necessarily vanish on all elements of the ideal boundary
, unlike the case of a hyperbolic finite Riemann surface, does not necessarily vanish on all elements of the ideal boundary 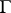 . The class
. The class 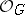 is also characterized as the class of Riemann surfaces with ideal boundary of zero capacity or, for short, as the class of Riemann surfaces with zero boundary. If
is also characterized as the class of Riemann surfaces with ideal boundary of zero capacity or, for short, as the class of Riemann surfaces with zero boundary. If  , then
, then 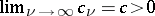 is called the capacity of the ideal boundary. The existence or non-existence of the Green function of a Riemann surface
is called the capacity of the ideal boundary. The existence or non-existence of the Green function of a Riemann surface 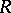 and also the contents of other function classes on
and also the contents of other function classes on 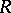 are determined first of all by this and other more subtle characteristics of the ideal boundary related to the function classes themselves.
are determined first of all by this and other more subtle characteristics of the ideal boundary related to the function classes themselves.
The principal function classes 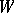 on a Riemann surface
on a Riemann surface 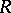 are the following:
are the following:
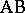 — the class of bounded single-valued analytic functions on
— the class of bounded single-valued analytic functions on 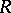 ;
;
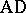 — the class of single-valued analytic functions
— the class of single-valued analytic functions 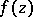 with a finite Dirichlet integral on
with a finite Dirichlet integral on 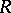 :
:
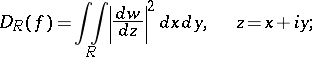 |
 ,
, 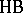 and
and  — the classes of single-valued harmonic functions on
— the classes of single-valued harmonic functions on 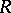 with, respectively, a positive, a bounded and a finite Dirichlet integral. These classes can be combined; for example,
with, respectively, a positive, a bounded and a finite Dirichlet integral. These classes can be combined; for example, 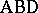 is the class of bounded single-valued analytic functions with a finite Dirichlet integral on
is the class of bounded single-valued analytic functions with a finite Dirichlet integral on 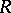 . For the corresponding classes
. For the corresponding classes 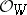 of
of 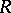 the following strict inclusions and equalities have been established:
the following strict inclusions and equalities have been established:
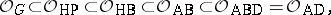 |
 |
For domains 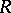 in the plane, these relations can be simplified:
in the plane, these relations can be simplified:
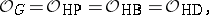 |
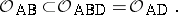 |
Of great importance are also the Hardy classes 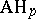 ,
, 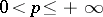 , of single-valued analytic functions
, of single-valued analytic functions 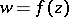 on
on 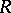 . For
. For  , a function
, a function  if the subharmonic function
if the subharmonic function 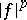 has a harmonic majorant on the entire Riemann surface
has a harmonic majorant on the entire Riemann surface 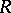 , and
, and 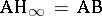 (see Boundary properties of analytic functions).
(see Boundary properties of analytic functions).
A Riemann surface of parabolic type is of class  , therefore the problem of characterizing Riemann surfaces of class
, therefore the problem of characterizing Riemann surfaces of class  is sometimes called the generalized problem of types. There are many results in which the condition that a Riemann surface belongs to the above-mentioned classes is established in different terms. Deep studies were dedicated to finding out the intrinsic properties of Riemann surfaces of the given classes. In particular, it turned out that Riemann surfaces with zero boundary are in many respects analogous to closed Riemann surfaces. The analogues of Abelian differentials (cf. Abelian differential) and the corresponding integrals can be constructed on them.
is sometimes called the generalized problem of types. There are many results in which the condition that a Riemann surface belongs to the above-mentioned classes is established in different terms. Deep studies were dedicated to finding out the intrinsic properties of Riemann surfaces of the given classes. In particular, it turned out that Riemann surfaces with zero boundary are in many respects analogous to closed Riemann surfaces. The analogues of Abelian differentials (cf. Abelian differential) and the corresponding integrals can be constructed on them.
More subtle properties of the ideal boundary of a Riemann surface  can be studied also by different compactifications of
can be studied also by different compactifications of  . For example, let
. For example, let  be the Wiener algebra of functions
be the Wiener algebra of functions  on a Riemann surface
on a Riemann surface  that are bounded, continuous and harmonizable on
that are bounded, continuous and harmonizable on  ; the latter means that for any regular domain
; the latter means that for any regular domain 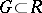 there exists a generalized solution of the Dirichlet problem in the sense of Wiener–Perron (see Perron method) with boundary values
there exists a generalized solution of the Dirichlet problem in the sense of Wiener–Perron (see Perron method) with boundary values  on the boundary
on the boundary 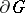 . The Wiener compactification of
. The Wiener compactification of 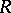 is the compact Hausdorff space
is the compact Hausdorff space  such that
such that  is an open dense subspace of
is an open dense subspace of 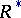 , each function
, each function 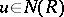 can be continuously continued onto
can be continuously continued onto 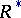 and
and 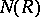 separates the points of
separates the points of  . The Wiener compactification exists for any Riemann surface
. The Wiener compactification exists for any Riemann surface 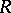 . The set
. The set 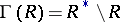 is called the Wiener ideal boundary of
is called the Wiener ideal boundary of  , and the subset
, and the subset 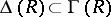 of those points of
of those points of 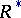 at which all potentials from
at which all potentials from 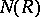 vanish, is called the Wiener harmonic boundary. In these terms, for example, the inclusion
vanish, is called the Wiener harmonic boundary. In these terms, for example, the inclusion 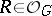 is equivalent to the equality
is equivalent to the equality 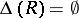 ; from this the strict inclusion
; from this the strict inclusion  follows also.
follows also.
The problem of removable sets (cf. Removable set) on Riemann surfaces is also related to the classification of Riemann surfaces. Thus, a compactum 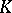 on a Riemann surface
on a Riemann surface 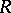 is called
is called 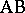 -removable if for some neighbourhood
-removable if for some neighbourhood 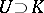 on
on 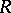 all
all 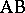 -functions on
-functions on 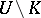 have an analytic continuation to the entire neighbourhood
have an analytic continuation to the entire neighbourhood  .
.
The attention of many scientists was also attracted by the problems of the classification of Riemannian manifolds of arbitrary dimensions 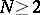 , related to the studies of the classes of functions described above.
, related to the studies of the classes of functions described above.
For references see Riemann surface.
Comments
In addition to the references quoted in Riemann surface and Riemann surfaces, conformal classes of, see also [a1]–[a2].
References
| [a1] | M. Tsuji, "Potential theory in modern function theory" , Chelsea, reprint (1975) |
| [a2] | C. Constantinescu, A. Cornea, "Ideale Ränder Riemannscher Flächen" , Springer pp. 1963 |
Riemann surfaces, classification of. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Riemann_surfaces,_classification_of&oldid=15266