Algebraic varieties, arithmetic of
arithmetical algebraic geometry
The branch of algebraic geometry in which one studies properties of algebraic varieties defined over fields of so-called arithmetic type, i.e. finite, local and global fields of algebraic numbers or algebraic functions. In the case of a finite field, its main subject is the study of the number of rational points of the algebraic variety in this field and in finite extensions of it. The zeta-function of the variety which is used in these studies strongly influenced the development of the methods of algebraic geometry. Estimates from below of the number of (rational) points [1], [4] are also important.
If  is an algebraic variety (or scheme) over a local field
is an algebraic variety (or scheme) over a local field 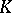 with field of residues
with field of residues 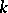 , then the study of the set
, then the study of the set  of rational points with values in
of rational points with values in 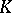 combines two different problems: To find solutions of congruences (or points of the variety over a finite field) and to find integral or rational solutions of Diophantine equations (cf. Hasse principle). If the variety
combines two different problems: To find solutions of congruences (or points of the variety over a finite field) and to find integral or rational solutions of Diophantine equations (cf. Hasse principle). If the variety 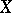 is defined by a set of equations with coefficients from the ring
is defined by a set of equations with coefficients from the ring 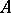 of integers of the field
of integers of the field 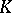 , then it is possible to define the reduction of this variety by the same set of equations, but with coefficients taken modulo the maximal ideal of
, then it is possible to define the reduction of this variety by the same set of equations, but with coefficients taken modulo the maximal ideal of 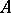 . A "variety"
. A "variety" 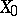 over the field of residues
over the field of residues 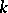 and a canonical mapping, or reduction,
and a canonical mapping, or reduction,
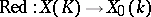 |
are obtained. This description of the reduction is difficult to explain in terms of classical algebraic geometry. This was one of the reasons for the introduction of the concept of a scheme — a language suitable for a rigorous description of the process. The main problem is to determine the image of the mapping Red, i.e. to find the points 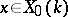 that come from the rational
that come from the rational 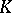 -points of the variety. The Hensel lemma states that
-points of the variety. The Hensel lemma states that  is such a point if
is such a point if  is a non-singular point. For more general results on this subject see [4].
is a non-singular point. For more general results on this subject see [4].
Another type of problems concerned with the local arithmetic of algebraic varieties is the study of forms over such fields. Let 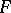 be a form in
be a form in  variables of degree
variables of degree 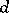 over a local field; Artin's conjecture is that if
over a local field; Artin's conjecture is that if 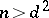 , then the equation
, then the equation 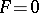 has a non-trivial solution. It is known that this statement is true in the case of function fields. It was proved for
has a non-trivial solution. It is known that this statement is true in the case of function fields. It was proved for 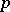 -adic fields that for each
-adic fields that for each  there exists a finite number
there exists a finite number 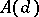 of primes such that Artin's conjecture holds for forms of degree
of primes such that Artin's conjecture holds for forms of degree 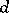 , if
, if 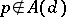 . It was shown in 1966 that the set
. It was shown in 1966 that the set 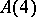 is non-empty, which proved Artin's conjecture to be false [4]. It is not yet (1977) known whether or not it is valid for forms of odd degree.
is non-empty, which proved Artin's conjecture to be false [4]. It is not yet (1977) known whether or not it is valid for forms of odd degree.
The arithmetic of algebraic varieties over global fields is the largest and most diverse part of algebraic geometry. It includes Diophantine geometry, class field theory, the theory of zeta-functions of varieties, and complex multiplication of Abelian functions (or varieties). All these theories were developed in parallel for number and function fields. Such a possibility was first demonstrated by the development of class field theory in the 1930s; it is based on the rough analogy between these fields which is most clearly manifested in the construction of the theory of schemes.
References
| [1] | Z.I. Borevich, I.R. Shafarevich, "Number theory" , Acad. Press (1966) (Translated from Russian) (German translation: Birkhäuser, 1966) |
| [2] | A. Weil, "Number theory and algebraic geometry" , Proc. Internat. Congress Mathematicians (Cambridge, 1950) , 2 , Amer. Math. Soc. (1952) pp. 90–100 |
| [3] | A. Grothendieck, J. Dieudonné, "Eléments de géometrie algébrique" Publ. Math. IHES , 4;8;11;17;20;24;28;32 |
| [4] | A.N. Parshin, "Arithmetic on algebraic varieties" J. Soviet Math. , 1 : 5 (1973) pp. 594–620 Itogi Nauk. Algebra Topol. Geom. 1970 , 1 (1970) pp. 111–152 |
| [5] | H.P.F. Swinnerton-Dyer, "Applications of algebraic geometry to number theory" , Proc. 1969 summer inst. number theory , Proc. Symp. Pure Math. , 20 , Amer. Math. Soc. (1971) pp. 1–52 |
Algebraic varieties, arithmetic of. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Algebraic_varieties,_arithmetic_of&oldid=15195