M-dependent-process
From Encyclopedia of Mathematics
A discrete-time stochastic process  is
is  -dependent if for all
-dependent if for all  the joint stochastic variables
the joint stochastic variables  are independent of the joint stochastic variables
are independent of the joint stochastic variables  .
.
Such processes arise naturally as limits of rescaling transformations (renormalizations) and (hence) as examples of processes with scaling symmetries [a1]. Examples of  -dependent processes are given by
-dependent processes are given by  -block factors. These are defined as follows. Let
-block factors. These are defined as follows. Let 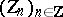 be an independent process and
be an independent process and 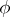 a function of
a function of 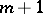 variables; let
variables; let 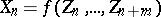 ; then the
; then the  -block factor
-block factor 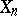 is an
is an 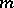 -dependent process.
-dependent process.
There are one-dependent processes which are not  -block factors, [a2].
-block factors, [a2].
References
| [a1] | G.L. O'Brien, "Scaling transformations for 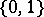 -valued sequences" Z. Wahrscheinlichkeitstheorie Verw. Gebiete , 53 (1980) pp. 35–49 -valued sequences" Z. Wahrscheinlichkeitstheorie Verw. Gebiete , 53 (1980) pp. 35–49 |
| [a2] | J. Aaronson, D. Gilat, M. Keane, V. de Valk, "An algebraic construction of a class of one-dependent processes" Ann. Probab. , 17 (1988) pp. 128–143 |
| [a3] | S. Janson, "Runs in 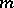 -dependent sequences" Ann. Probab. , 12 (1984) pp. 805–818 -dependent sequences" Ann. Probab. , 12 (1984) pp. 805–818 |
| [a4] | G. Haiman, "Valeurs extrémales de suites stationaires de variable aléatoires 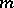 -dépendantes" Ann. Inst. H. Poincaré Sect. B (N.S.) , 17 (1981) pp. 309–330 -dépendantes" Ann. Inst. H. Poincaré Sect. B (N.S.) , 17 (1981) pp. 309–330 |
How to Cite This Entry:
M-dependent-process. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=M-dependent-process&oldid=14932
M-dependent-process. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=M-dependent-process&oldid=14932