A tensor describing the locations of the points of a body after deformation with respect to their location before deformation. It is a symmetric tensor of the second rank,
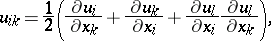 | (*) |
where  are the Cartesian rectangular coordinates of a point in the body prior to deformation and
are the Cartesian rectangular coordinates of a point in the body prior to deformation and 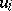 are the coordinates of the displacement vector
are the coordinates of the displacement vector 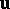 . In the theory of elasticity the deformation tensor is decomposed into two constituent tensors:
. In the theory of elasticity the deformation tensor is decomposed into two constituent tensors:
The tensor  describes a spatial deformation and is known as the spherical deformation tensor:
describes a spatial deformation and is known as the spherical deformation tensor:
The tensor  describes solely the change in form, and the sum of its diagonal elements is equal to zero:
describes solely the change in form, and the sum of its diagonal elements is equal to zero:
The tensor 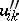 is known as the deviator of the deformation tensor.
is known as the deviator of the deformation tensor.
In the case of a small deformation, second-order magnitudes are neglected, and the deformation tensor (*) is defined by the expression:
In spherical coordinates  the linearized deformation tensor (*) assumes the form:
the linearized deformation tensor (*) assumes the form:
In cylindrical coordinates 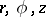 it has the form
it has the form
References
| [1] | L.D. Landau, E.M. Lifshits, "Theory of elasticity" , Pergamon (1959) (Translated from Russian) |
| [2] | , [Soviet] Physical Encyclopedic Dictionary , 1 , Moscow (1960) pp. 553 (In Russian) |
Strictly speaking the  of (*) do not form a tensor in the mathematical sense; they do not transform as tensors. The "tensor"
of (*) do not form a tensor in the mathematical sense; they do not transform as tensors. The "tensor"  is also known as the strain tensor or the rate-of-strain tensor.
is also known as the strain tensor or the rate-of-strain tensor.
Let  and
and  denote line elements (infinitesimal distances) before and after deformation. Then
denote line elements (infinitesimal distances) before and after deformation. Then  , so that
, so that  describes the change in an element of length as the body is deformed.
describes the change in an element of length as the body is deformed.
References
| [a1] | A.C. Eringen, "Mechanics of continua" , Pergamon (1967) |
How to Cite This Entry:
Deformation tensor. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Deformation_tensor&oldid=14896
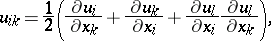
 are the Cartesian rectangular coordinates of a point in the body prior to deformation and
are the Cartesian rectangular coordinates of a point in the body prior to deformation and 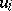 are the coordinates of the displacement vector
are the coordinates of the displacement vector 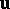 . In the theory of elasticity the deformation tensor is decomposed into two constituent tensors:
. In the theory of elasticity the deformation tensor is decomposed into two constituent tensors:
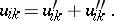
 describes a spatial deformation and is known as the spherical deformation tensor:
describes a spatial deformation and is known as the spherical deformation tensor:
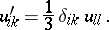
 describes solely the change in form, and the sum of its diagonal elements is equal to zero:
describes solely the change in form, and the sum of its diagonal elements is equal to zero:
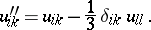
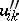 is known as the deviator of the deformation tensor.
is known as the deviator of the deformation tensor.

 the linearized deformation tensor (*) assumes the form:
the linearized deformation tensor (*) assumes the form:

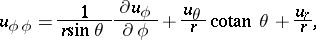

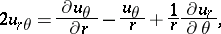

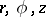 it has the form
it has the form

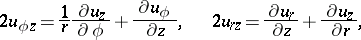
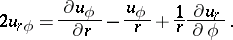
 of (*) do not form a tensor in the mathematical sense; they do not transform as tensors. The "tensor"
of (*) do not form a tensor in the mathematical sense; they do not transform as tensors. The "tensor"  is also known as the strain tensor or the rate-of-strain tensor.
is also known as the strain tensor or the rate-of-strain tensor.
 and
and  denote line elements (infinitesimal distances) before and after deformation. Then
denote line elements (infinitesimal distances) before and after deformation. Then  , so that
, so that  describes the change in an element of length as the body is deformed.
describes the change in an element of length as the body is deformed.