Bell numbers
From Encyclopedia of Mathematics
The Bell numbers  are given by
are given by
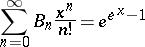 |
or by
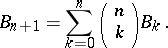 |
Also,
 |
where  are Stirling numbers (cf. Combinatorial analysis) of the second kind, so that
are Stirling numbers (cf. Combinatorial analysis) of the second kind, so that  is the total number of partitions of an
is the total number of partitions of an  -set.
-set.
They are equal to  .
.
The name honours E.T. Bell.
References
| [a1] | L. Comtet, "Advanced combinatorics" , Reidel (1974) |
How to Cite This Entry:
Bell numbers. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bell_numbers&oldid=14335
Bell numbers. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bell_numbers&oldid=14335
This article was adapted from an original article by N.J.A. Sloane (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article