Faddeev equation

A linear integral equation of quantum mechanics describing the scattering of three particles.

The scattering of three particles differs fundamentally from that of two in that it is possible to form bound states of the particles. Therefore the usual radiation condition at infinity of the type of the Sommerfeld condition cannot be applied here.

The mathematical investigation of three-particle systems became possible after L.D. Faddeev in 1960 proposed and studied an integral equation via the solutions of which the solutions of the Schrödinger equation satisfying appropriate physical conditions at infinity can be recovered.

In abbreviated vector notation the Faddeev equation has the form:

(*)

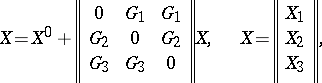where 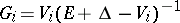, 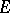is the energy of the system, the 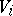are the potentials of interactions of pairs of particles, and the vector function 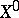is determined by the initial data of the scattering. If the scattering problem is formulated in terms of the Schrödinger equation with right-hand side 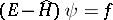, where 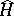is the three-particle Hamiltonian

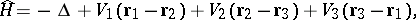then one must take 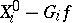in (*). Then the solution 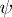of the scattering problem can be expressed in terms of the solution 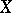of the Faddeev equation by the formula

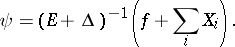Under suitable conditions on the potentials 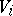, equation (*) is of Fredholm type (see [1]). Moreover, equation (*) is used to prove the theorem on eigen function expansion of the Schrödinger operator, to give a foundation for the non-stationary formulation of the scattering problem, and to construct a unitary scattering operator.

The Faddeev equation is widely applied in atomic and nuclear physics and in elementary particle physics. A relativistic version of it has been obtained and also a generalization to the case of a system of 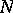particles. An important advantage of the Faddeev equation compared with the Schrödinger equation is that it is possible to compute the solution effectively.

References

[1]	L.D. Faddeev, "Mathematical aspects of the three-body problem in the quantum scattering theory" , Israel Program Sci. Transl. (1965) (Translated from Russian)
[2]	E. Schmid, H. Ziegelmann, "The quantum-mechanical three-body problem" , Braunschweig (1974)

References

[a1]	Ferreira (ed.) et al. (ed.) , Models and methods in few body physics. Proc. Lisboa 1986 , Springer
[a2]	T.K. Lim (ed.) , Few body methods, principles and applications , World Sci. (1985)
[a3]	"Few body systems in particle and nuclear physics. Proc. 11-th Conf. Tokyo, August 1986" Nuclear Physics , A 463 : 1–2 (1987)