Complex (in homological algebra)
One of the basic concepts of homological algebra. Let  be an Abelian category. A graded object is a sequence
be an Abelian category. A graded object is a sequence  of objects
of objects  in
in  . A sequence
. A sequence  of morphisms
of morphisms  is called a morphism
is called a morphism  of graded objects. One defines the object
of graded objects. One defines the object  by setting
by setting  . A morphism of graded objects
. A morphism of graded objects 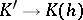 is called a morphism of degree
is called a morphism of degree  from
from  into
into  . A graded object is said to be positive if
. A graded object is said to be positive if  for all
for all  , bounded from below if
, bounded from below if 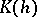 is positive for some
is positive for some  and finite or bounded if
and finite or bounded if  for all but a finite number of integers
for all but a finite number of integers  . A chain complex in a category
. A chain complex in a category 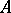 consists of a graded object
consists of a graded object  and a morphism
and a morphism  of degree
of degree  such that
such that  . More precisely:
. More precisely: 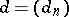 , where
, where  and
and 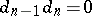 for any
for any  . A morphism of chain complexes
. A morphism of chain complexes
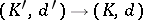 |
is a morphism  of graded objects for which
of graded objects for which  . A cochain complex is defined in a dual manner (as a graded object with a morphism
. A cochain complex is defined in a dual manner (as a graded object with a morphism  of degree
of degree  ).
).
Most frequently, complexes are considered in categories of Abelian groups, modules or sheaves of Abelian groups on a topological space. Thus, a complex of Abelian groups is a graded differential group the differential of which has degree 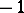 or
or  .
.
Associated with each complex 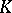 are the three graded objects:
are the three graded objects:
the boundaries  , where
, where  ;
;
the cycles  , where
, where 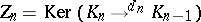 ; and
; and
the  -dimensional homology objects (classes)
-dimensional homology objects (classes) 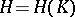 , where
, where  (see Homology of a complex).
(see Homology of a complex).
For a cochain complex, the analogous objects are called coboundaries, cocycles and cohomology objects (notations 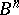 ,
,  and
and 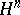 , respectively).
, respectively).
If 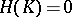 , then the complex
, then the complex  is said to be acyclic.
is said to be acyclic.
A morphism  of complexes induces morphisms
of complexes induces morphisms
 |
and hence a homology or cohomology morphism
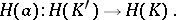 |
Two morphisms  are said to be homotopic (denoted by
are said to be homotopic (denoted by 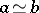 ) if there is a morphism
) if there is a morphism 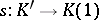 (or
(or  for cochain complexes) of graded objects (called a homotopy), such that
for cochain complexes) of graded objects (called a homotopy), such that
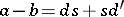 |
(which implies that  ). A complex
). A complex  is said to be contractible if
is said to be contractible if  , in which case the complex
, in which case the complex  is acyclic.
is acyclic.
If  is an exact sequence of complexes, then there exists a connecting morphism
is an exact sequence of complexes, then there exists a connecting morphism  of degree
of degree  (
( ) that is natural with respect to morphisms of exact sequences and is such that the long homology sequence (that is, the sequence
) that is natural with respect to morphisms of exact sequences and is such that the long homology sequence (that is, the sequence
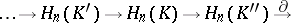 |
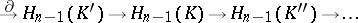 |
for a chain complex, and the sequence
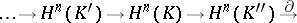 |
 |
for a cochain complex) is exact.
The cone of a morphism 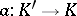 of chain complexes is the complex
of chain complexes is the complex  defined as follows:
defined as follows:
 |
with
 |
The direct sum decomposition of the complex  leads to an exact sequence of complexes
leads to an exact sequence of complexes
 |
for which the associated long homology sequence is isomorphic to the sequence
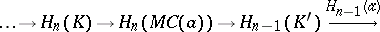 |
 |
Hence the chain complex  is acyclic if and only if
is acyclic if and only if  is an isomorphism. Analogous notions and facts hold for cochain complexes.
is an isomorphism. Analogous notions and facts hold for cochain complexes.
References
| [1] | H. Bass, "Algebraic  -theory" , Benjamin (1968) -theory" , Benjamin (1968) |
| [2] | H. Cartan, S. Eilenberg, "Homological algebra" , Princeton Univ. Press (1956) |
| [3] | P.J. Hilton, U. Stammbach, "A course in homological algebra" , Springer (1971) |
| [4] | S. MacLane, "Homology" , Springer (1963) |
Complex (in homological algebra). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Complex_(in_homological_algebra)&oldid=14272