Demoulin theorem
From Encyclopedia of Mathematics
A helicoid has an infinite number (viz.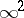 
) of systems of conjugate nets of lines which are preserved under continuous deformation of this surface — its principal bases (cf. Deformation over a principal base). Established by A. Demoulin [1]. It then turns out that these principal bases are Voss nets (cf. Voss net). Conversely (Finikov's theorem), the only surface with an infinite number of principal bases is a right helicoid [2].
References
| [1] | A. Demoulin, "Sur les systèmes conjugués persistants" C.R. Acad. Sci. Paris , 133 (1901) pp. 986–990 |
| [2] | S.P. Finikov, "Bending and related geometrical problems" , Moscow-Leningrad (1937) (In Russian) |
How to Cite This Entry:
Demoulin theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Demoulin_theorem&oldid=13871
Demoulin theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Demoulin_theorem&oldid=13871
This article was adapted from an original article by M.I. Voitsekhovskii (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article