Weak homology
An equivalence relation between cycles leading to the definition of the spectral homology groups 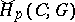 . It is known that the Steenrod–Sitnikov homology groups
. It is known that the Steenrod–Sitnikov homology groups 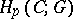 of a compact space map epimorphically onto
of a compact space map epimorphically onto 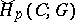 , and that the kernel
, and that the kernel 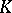 of this epimorphism is isomorphic to the first derived functor
of this epimorphism is isomorphic to the first derived functor 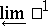 of the inverse limit of the homology groups
of the inverse limit of the homology groups  of the nerves of the open coverings
of the nerves of the open coverings 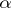 of the space
of the space 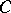 . The groups
. The groups 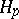 were originally defined in terms of Vietoris cycles, and the cycles giving the elements of the subgroup
were originally defined in terms of Vietoris cycles, and the cycles giving the elements of the subgroup  were called weakly homologous to zero. On the other hand, Vietoris cycles homologous to zero in the above definition of the groups
were called weakly homologous to zero. On the other hand, Vietoris cycles homologous to zero in the above definition of the groups 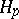 are sometimes called strongly homologous to zero (and the corresponding equivalence relation between them is called strong homology). In the case when
are sometimes called strongly homologous to zero (and the corresponding equivalence relation between them is called strong homology). In the case when  is a compact group or a field, the kernel
is a compact group or a field, the kernel 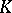 is equal to zero, and the concepts of strong and weak homology turn out to be equivalent.
is equal to zero, and the concepts of strong and weak homology turn out to be equivalent.
References
| [1] | P.S. Aleksandrov, "Topological duality theorems II. Non-closed sets" Trudy Mat. Inst. Steklov. , 54 (1959) pp. 3–136 (In Russian) |
| [2] | W.S. Massey, "Notes on homology and cohomology theory" , Yale Univ. Press (1964) |
Weak homology. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Weak_homology&oldid=13822