Comparison theorem (algebraic geometry)
A theorem on the relations between homotopy invariants of schemes of finite type over the field  in classical and étale topologies.
in classical and étale topologies.
Let  be a scheme of finite type over
be a scheme of finite type over  , while
, while  is a constructible torsion sheaf of Abelian groups on
is a constructible torsion sheaf of Abelian groups on  . Then
. Then  induces a sheaf on
induces a sheaf on  in the classical topology, and there exist canonical isomorphisms
in the classical topology, and there exist canonical isomorphisms
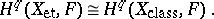 |
On the other hand, a finite topological covering of a smooth scheme  of finite type over
of finite type over  has a unique algebraic structure (Riemann's existence theorem). The fundamental étale group of
has a unique algebraic structure (Riemann's existence theorem). The fundamental étale group of 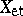 [1] is therefore the pro-finite completion of the ordinary group of classes of homotopically equivalent loops:
[1] is therefore the pro-finite completion of the ordinary group of classes of homotopically equivalent loops:
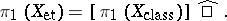 |
Moreover, if 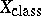 is simply connected, then
is simply connected, then 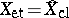 , where
, where 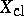 and
and 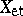 are the classical and étale homotopy types of the scheme
are the classical and étale homotopy types of the scheme  , respectively (see [1], [2]).
, respectively (see [1], [2]).
References
| [1] | M. Artin, "The étale topology of schemes" , Proc. Internat. Congress Mathematicians (Moscow, 1966) , Mir (1968) pp. 44–56 |
| [2] | D. Sullivan, "Geometric topology" , M.I.T. (1971) (Notes) |
Comparison theorem (algebraic geometry). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Comparison_theorem_(algebraic_geometry)&oldid=13795