Banach limit

Banach limits originated in [a1], Chapt. II, Sect. 3. Denoting the positive integers by , the set is the real vector space of all bounded sequences of real numbers. For any element , one defines by for all 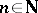. S. Banach showed that there exists an element in the dual , called , such that

1) for all ;

2) 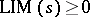for all non-negative sequences ;

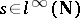3) 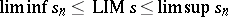for all ;

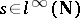4) 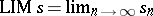for all convergent sequences 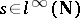. Banach proved the existence of this generalized limit by using the Hahn–Banach theorem. Today (1996), Banach limits are studied via the notion of amenability.

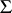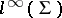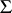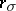For a semi-group one defines to be the real vector space of all real bounded functions on . For an element one denotes the left (respectively, right) shift by (respectively, ). Thus, 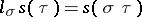for all and 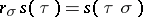for all 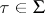. An element is called a left- (respectively right-) invariant mean if

1) ;

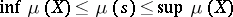2) 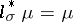(respectively, ), where, e.g., 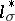is the adjoint of . itself is called left (respectively, right) amenable if there exists a left- (respectively, right-) invariant mean in 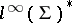. The existence of Banach limits above is a special case of an invariant mean, where equals the semi-group of natural numbers. Banach also proved that the real numbers are amenable (left and right). M.M. Day has proved that every Abelian semi-group is left and right amenable. On the other hand, , the free group on two generators, is not amenable.

Another approach to amenability is the measure-theoretic point of view. In fact, the prehistory of amenability starts with the following question by H. Lebesgue in the classic "Leçons sur L'Intégration et la Recherche des Fonctions Primitives" ([a5], pp. 114–115): Can countable additivity of the Lebesgue measure be replaced by finite additivity? Banach answered the question in the negative, constructing a finitely additive measure on all subsets of the real numbers, invariant under translation, again using the Hahn–Banach theorem. More generally, if a group 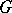is acting on a set 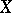, a finitely additive probability measure on the collection of all subsets of 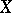, invariant under 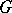, is sometimes also called an invariant mean. If 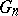is the isometry group of 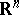, one can ask for a finitely additive measure invariant under 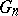. Such a measure does exist for 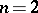, but not 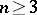for . For 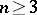this leads to so-called paradoxical decompositions or the Banach–Tarski paradox (see Tarski problem; for a survey, see [a8]). For all 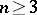, the group contains the non-amenable as a subgroup. It has been proved that the Banach–Tarski paradox is effectively (i.e., in ZF set theory) implied by the Hahn–Banach theorem (see [a7]).

For a survey of results of the role of amenability, see [a6] and for a survey of the Hahn–Banach theorem, see [a2]. For the early history of Banach limits and invariant means, including many important results, see [a3] and [a4].

References

[a1]	S. Banach, "Théorie des opérations linéaires" , PWN (1932)
[a2]	G. Buskes, "The Hahn–Banach theorem surveyed" Dissertationes Mathematicae , CCCXXVII (1993)
[a3]	M.M. Day, "Normed linear spaces" , Ergebnisse der Mathematik und ihrer Grenzgebiete , 21 , Springer (1973)
[a4]	Greenleaf, F.P, "Invariant means on topological groups and their applications" , v. Nostrand (1969)
[a5]	H. Lebesgue, "Oeuvres Scientifiques" , L'Enseign. Math. , II , Inst. Math. Univ. Genæve (1972)
[a6]	A.L.T. Paterson, "Amenibility" , Mathematical Surveys and Monographs , 29 , Amer. Math. Soc. (1988)
[a7]	J. Pawlikowski, "The Hahn–Banach theorem implies the Banach–Tarski paradox" Fundam. Math. , 138 (1991) pp. 20–22
[a8]	S. Wagon, "The Banach–Tarski paradox" , Cambridge Univ. Press (1986)