Final object

terminal object, of a category

A concept formalizing the categorical property of a one-point set. An object in a category is called final if for every object in the set consists of a single morphism. A final object is also called a right null object of . A left null or initial object of a category is defined in the dual way.

In the category of sets the final objects are just the one-point sets. In any category with null objects the final objects are the null objects. Other examples of final objects arise in various categories of diagrams, where the concept of a final object is essentially equivalent to that of the limit of a diagram. 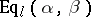For example, suppose that and let be the category of left equalizers of the pair ; in other words, the objects of are morphisms for which , and morphisms in are morphisms for which 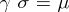. A final object in 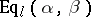is a kernel of the pair of morphisms 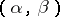(cf. Kernel of a morphism in a category).

Comments

The set 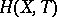is, by definition, the set of morphisms 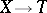. Note that any two final objects of a category are (canonically) isomorphic, and so are two initial objects.

References

[a1]	J. Adámek, "Theory of mathematical structures" , Reidel (1983)
[a2]	B. Mitchell, "Theory of categories" , Acad. Press (1965) pp. 4