Stirling formula

An asymptotic representation which provides approximate values of the factorials and of the gamma-function for large values of . This representation has the form

(*)

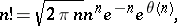where 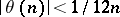. The asymptotic equalities

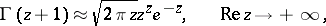hold, and mean that when 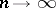or 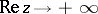, the ratio of the left- and right-hand sides tends to one.

The representation (*) was established by J. Stirling (1730).

Comments

See Gamma-function for the corresponding asymptotic series (Stirling series) and additional references.

References

[a1]	N.G. de Bruijn, "Asymptotic methods in analysis" , Dover, reprint (1981)
[a2]	G. Marsaglia, J.C.W. Marsaglia, "A new derivation of Stirling's approximation of " Amer. Math. Monthly , 97 (1990) pp. 826–829
[a3]	V. Namias, "A simple derivation of Stirling's asymptotic series" Amer. Math. Monthly , 93 (1986) pp. 25–29