Laplace transformation (in geometry)
From Encyclopedia of Mathematics
The transition from one focal net of a congruence to another focal net of the same congruence. The concept of the Laplace transformation of a net was introduced by G. Darboux (1888), who discovered that an analytic transformation of the solutions of the Laplace equation
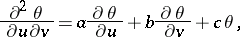 |
where  ,
, 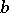 ,
,  are known functions of the variables
are known functions of the variables  ,
,  , can be interpreted geometrically as transition from one focal net of a congruence to another focal net of it. The Laplace transformation of nets establishes a correspondence between the theory of conjugate nets (cf. Conjugate net) and line geometry. There are various generalizations of the Laplace transformation of a net.
, can be interpreted geometrically as transition from one focal net of a congruence to another focal net of it. The Laplace transformation of nets establishes a correspondence between the theory of conjugate nets (cf. Conjugate net) and line geometry. There are various generalizations of the Laplace transformation of a net.
References
| [1] | G. Tzitzeica, "Géométrie différentielle projective des réseaux" , Gauthier-Villars & Acad. Roumaine (1924) |
| [2] | V.T. Bazylev, "Multidimensional nets and their transformations" Itogi Nauk. Geom. 1963 (1965) pp. 138–164 (In Russian) |
Comments
Cf. also Laplace sequence.
References
| [a1] | S.P. Finikov, "Theorie der Kongruenzen" , Akademie Verlag (1959) (Translated from Russian) |
How to Cite This Entry:
Laplace transformation (in geometry). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Laplace_transformation_(in_geometry)&oldid=13530
Laplace transformation (in geometry). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Laplace_transformation_(in_geometry)&oldid=13530
This article was adapted from an original article by V.T. Bazylev (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article