Dupin cyclide
A surface for which both families of curvature lines consist of circles, so that it is a special case of a canal surface. Both sheets of the focal set of a Dupin cyclide degenerate to curves,  and
and  , which are curves of the second order. There are three types of Dupin cyclides.
, which are curves of the second order. There are three types of Dupin cyclides.
1) The evolutes are an ellipse and a hyperbola; the radius vector of the corresponding Dupin cyclide is
 |
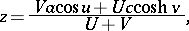 |
where
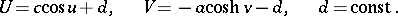 |
2) The evolutes are focal parabolas; the radius vector is
 |
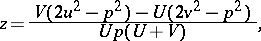 |
where
 |
3) The evolutes are a circle and a straight line; the corresponding Dupin cyclide is a torus.
Dupin cyclides are algebraic surfaces of order four in the cases 1) and 3) above, and of order three in the case 2).
References
| [1] | Ch. Dupin, "Développements de géométrie" , Paris (1813) |
| [2] | F. Klein, "Vorlesungen über höhere Geometrie" , Springer (1926) |
| [3] | D. Hilbert, S.E. Cohn-Vossen, "Anschauliche Geometrie" , Springer (1932) |
Comments
Originally, a Dupin cycle was defined more geometrically as the envelope of a family of spheres tangent to three fixed spheres. Every Dupin cyclide can be obtained from the following three examples by inversion in a suitable sphere: a torus of revolution, a circular cylinder and a circular cone.
The Dupin cyclides are surfaces of the second order in pentaspherical coordinates and have, moreover, two equal axis. For more on Dupin cyclides see [a2], pp. 359-360 and [a3], pp. 355-356; 441. A remarkable property of cyclides is the fact that they carry four families of circles.
The natural generalization of the Dupin cyclides to higher dimensions are the so-called Dupin-hypersurfaces (see [a1]).
References
| [a1] | T.E. Cecil, P.J. Ryan, "Tight and taut immersions of manifolds" , Pitman (1985) |
| [a2] | M. Berger, "Geometry" , II , Springer (1987) |
| [a3] | M. Berger, B. Gostiaux, "Differential geometry: manifolds, curves, and surfaces" , Springer (1988) (Translated from French) |
| [a4] | W. Blaschke, K. Leichtweiss, "Elementare Differentialgeometrie" , Springer (1973) |
Dupin cyclide. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Dupin_cyclide&oldid=13429