Bianchi identity

A relation connecting the components of the covariant derivatives of the curvature tensor 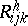of a Riemannian space:

where 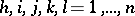. First established by L. Bianchi [1] in 1902.

References

[1]	L. Bianchi, "Lezioni di geometria differenziale" , 1–2 , Zanichelli , Bologna (1923–1927)

Comments

Here 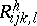denotes of course the covariant derivative of 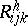with respect to the 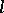-th coordinate.

The identity described above is often called the second Bianchi identity. The first Bianchi identity is then given by

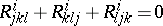(see [a1], [a2]). Generalized versions of these identities for curvature forms and curvature tensors of connections with torsion are given in [a2].

References

[a1]	N.J. Hicks, "Notes on differential geometry" , v. Nostrand (1965)
[a2]	S. Kobayashi, K. Nomizu, "Foundations of differential geometry" , 1 , Interscience (1963)