Riemann hypotheses

in analytic number theory

Five conjectures, formulated by B. Riemann (1876), concerning the distribution of the non-trivial zeros of the zeta-function , and the expression via these zeros of the number of prime numbers not exceeding a real number . One of the Riemann hypotheses has neither been proved nor disproved: All non-trivial zeros of the zeta-function 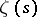lie on the straight line .

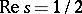Comments

For the list of all 5 conjectures see Zeta-function.

References

[a1]	A. Ivic, "The Riemann zeta-function" , Wiley (1985)
[a2]	E.C. Titchmarsh, "The theory of the Riemann zeta-function" , Clarendon Press (1951)
[a3]	H.M. Edwards, "Riemann's zeta function" , Acad. Press (1974) pp. Chapt. 3