Runge theorem

A theorem on the possibility of polynomially approximating holomorphic functions, first proved by C. Runge (1885) (cf. also Approximation of functions of a complex variable).

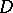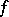Let be a simply-connected domain in the complex -plane. Then any function holomorphic in 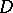can be approximated uniformly on compact sets inside 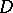by polynomials in . More precisely, for any compact set 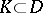and 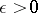there is a polynomial 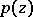with complex coefficients such that 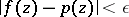for all .

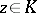In other words: Any function holomorphic in a simply-connected domain 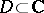can be represented as a series of polynomials in converging absolutely and uniformly to 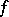on compact sets inside .

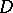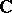An equivalent statement of Runge's theorem: Let be a compact set in with connected complement 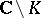; then any function holomorphic in a neighbourhood of 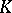can be approximated uniformly on 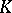by polynomials in . In this formulation, Runge's theorem is a special case of Mergelyan's theorem (cf. Mergelyan theorem).

The following theorem on rational approximation is also called Runge's theorem: Any function 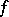holomorphic in a domain can be uniformly approximated on compact sets inside 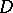by rational functions with poles outside .

Runge's theorem has many applications in the theory of functions of a complex variable and in functional analysis. A theorem analogous to Runge's theorem is valid for non-compact Riemann surfaces. An extension of Runge's theorem to functions of several complex variables is the Oka–Weil theorem (see Oka theorems).

References

[1]	A.I. Markushevich, "A short course on the theory of analytic functions" , Moscow (1978) (In Russian)
[2]	B.V. Shabat, "Introduction of complex analysis" , 1 , Moscow (1976) (In Russian)

Comments

For more about Runge's theorem and its generalizations, such as Walsh' theorem, the Keldysh theorem, the Lavrent'ev theorem, see [a6], [a1], Chapt. VIII, and [a3], Chapt. III, for the case of the complex plane; [a2], Sect. 25, for the case of Riemann surfaces; and [a4], Sect. 7, for the case of several complex variables.

References

[a1]	R.B. Burchel, "An introduction to classical complex analysis" , 1 , Acad. Press (1979)
[a2]	O. Forster, "Lectures on Riemann surfaces" , Springer (1981) (Translated from German)
[a3]	D. Gaier, "Lectures on complex approximation" , Birkhäuser (1987) (Translated from German)
[a4]	J. Wermer, "Banach algebras and several complex variables" , Springer (1976) pp. Chapt. 13
[a5]	W. Rudin, "Real and complex analysis" , McGraw-Hill (1978) pp. 24
[a6]	A.I. Markushevich, "Theory of functions of a complex variable" , 3 , Chelsea (1977) pp. Sect. 3.12 (Translated from Russian)