Suslin problem

Is a dense complete linearly ordered set without first and last elements, in which every family of non-empty disjoint intervals is countable, isomorphic to the set of real numbers?

The assertion that the answer to this question is positive is the Suslin hypothesis, proposed by M.Ya. Suslin [1]. The Suslin hypothesis is equivalent to the non-existence of a linearly ordered non-separable 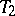-compactum in which every family of non-empty disjoint intervals is countable — such a 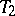-compactum is called a Suslin continuum, or Suslin line.

The Suslin problem is known to be independent of the fundamental axioms of set theory. A Suslin continuum was first constructed by means of the forcing method in 1967–1968. In 1970 it was proved that the conjunction of Martin's axiom and the negation of the continuum hypothesis (which is compatible with the Zermelo–Fraenkel system of axioms of set theory) implies the non-existence of a Suslin continuum, i.e. that the Suslin hypothesis holds.

References

[1]	M. [M.Ya. Suslin] Souslin, "Problème 3" Fundam. Mat. , 1 (1920) pp. 223

Comments

For more references see Suslin hypothesis.