Carleman boundary value problem

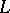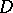A boundary value problem for analytic functions involving a shift which reverses the direction of traversing the boundary. It was first considered by T. Carleman [1]. Let be a simple closed Lyapunov curve (cf. Lyapunov surfaces and curves) in the complex -plane and let be the finite domain bounded by 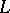. Let 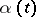be a given complex-valued function on 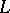inducing a one-to-one mapping of 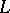onto itself reversing the direction of traversing 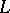and satisfying additionally the Carleman condition:

(*)

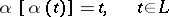(it is further supposed that the derivative 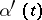satisfies a Hölder condition). Then the Carleman boundary value problem consists in finding a function , analytic in 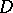except for a finite number of poles, continuous on 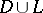and subject to the boundary condition

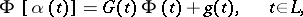where the functions 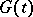and given on 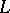satisfy a Hölder condition and 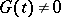on .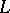

The Carleman boundary value problem has also been studied under the condition

which is more general than (*), and so has the Carleman boundary value problem for several unknown functions (see [2], [3]).

References

[1]	T. Carleman, "Sur la théorie des équations intégrales et ses applications" , Verh. Internat. Mathematiker Kongress. Zürich, 1932 , 1 , O. Füssli (1932) pp. 138–151
[2]	N.I. Muskhelishvili, "Singular integral equations" , Wolters-Noordhoff (1977) (Translated from Russian)
[3]	N.P. Vekua, "Systems of singular integral equations and some boundary value problems" , Moscow (1970) (In Russian)