Plücker interpretation
A model that realizes the geometry of the three-dimensional projective space 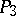 in the hyperbolic space
in the hyperbolic space 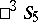 . The Plücker interpretation is based on a special interpretation of the Plücker coordinates of a straight line, which are defined for any straight line in
. The Plücker interpretation is based on a special interpretation of the Plücker coordinates of a straight line, which are defined for any straight line in 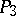 .
.
Under projective transformations of 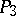 the Plücker coordinates transform linearly; the Plücker coordinates of straight lines in
the Plücker coordinates transform linearly; the Plücker coordinates of straight lines in  give a one-to-one correspondence between the straight lines of
give a one-to-one correspondence between the straight lines of 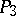 and the points in the projective space
and the points in the projective space 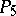 whose coordinates are numerically equal to the Plücker coordinates in
whose coordinates are numerically equal to the Plücker coordinates in 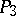 .
.
Straight lines in 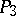 are represented by the points of a non-singular quadric in
are represented by the points of a non-singular quadric in 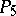 of index three.
of index three.
If one takes this quadric as the absolute and defines a projective (non-Euclidean) metric in 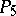 , one gets the five-dimensional hyperbolic space
, one gets the five-dimensional hyperbolic space 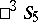 . Under each collineation and correlation of
. Under each collineation and correlation of 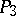 the Plücker coordinates transform linearly, i.e. each collineation and correlation is represented by a collineation of
the Plücker coordinates transform linearly, i.e. each collineation and correlation is represented by a collineation of 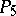 that maps the absolute into itself. These collineations are thus displacements of
that maps the absolute into itself. These collineations are thus displacements of 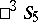 . The displacements of
. The displacements of 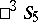 represent either collineations or correlations in
represent either collineations or correlations in 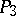 .
.
Each line complex in 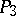 is put into correspondence with a point in
is put into correspondence with a point in 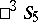 . The projective geometry of
. The projective geometry of 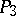 can be considered as a non-Euclidean geometry of
can be considered as a non-Euclidean geometry of 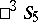 . This interpretation of the geometry of
. This interpretation of the geometry of 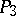 in
in 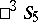 is called the Plücker interpretation, in connection with the role of the Plücker coordinates.
is called the Plücker interpretation, in connection with the role of the Plücker coordinates.
If one takes a straight line as the basic object in 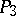 , the geometry of this space can be considered as the geometry on the absolute of
, the geometry of this space can be considered as the geometry on the absolute of 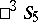 .
.
The group of projective transformations of 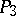 is isomorphic to the group of displacements of
is isomorphic to the group of displacements of 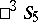 , and any involutory projective transformation of
, and any involutory projective transformation of 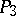 corresponds to an involutory displacement in
corresponds to an involutory displacement in 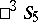 . For example, a null system in
. For example, a null system in 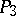 corresponds to a reflection in a point and its polar hyperplane in
corresponds to a reflection in a point and its polar hyperplane in 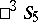 ; an involutory homology in
; an involutory homology in  corresponds to a hyperbolic paratactic displacement by a half-line in
corresponds to a hyperbolic paratactic displacement by a half-line in 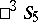 , etc. Each connected component of the group of projective transformations for
, etc. Each connected component of the group of projective transformations for 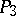 corresponds to a connected component of the group of displacements for
corresponds to a connected component of the group of displacements for 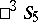 .
.
1) A collineation of 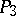 with positive determinant, including the identity transformation, corresponds to a displacement in
with positive determinant, including the identity transformation, corresponds to a displacement in 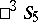 with determinant
with determinant 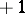 (identity transformations are included here).
(identity transformations are included here).
2) Any correlation in 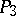 with positive determinant (including the null system) corresponds to a displacement in
with positive determinant (including the null system) corresponds to a displacement in 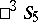 with determinant equal to
with determinant equal to 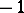 that transforms the proper and ideal domains, respectively, into themselves (including reflections in a point).
that transforms the proper and ideal domains, respectively, into themselves (including reflections in a point).
3) Any collineation of 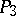 having negative determinant corresponds to a displacement in
having negative determinant corresponds to a displacement in 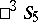 with determinant
with determinant 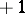 that transforms the proper domain into the ideal domain and vice versa, and this component contains a hyperbolic displacement by a half-line.
that transforms the proper domain into the ideal domain and vice versa, and this component contains a hyperbolic displacement by a half-line.
4) Any correlation in 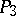 having negative determinant corresponds to a displacement in
having negative determinant corresponds to a displacement in 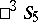 with determinant
with determinant 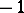 that transforms the proper domain into the ideal domain and vice versa.
that transforms the proper domain into the ideal domain and vice versa.
The images under symmetries that correspond to one another in 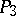 and
and 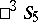 are put into correspondence with numerical invariants, between which there are certain relationships.
are put into correspondence with numerical invariants, between which there are certain relationships.
The Plücker interpretation is used in research on the displacement groups for the three-dimensional non-Euclidean spaces 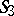 ,
, 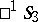 and
and 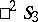 , which are isomorphic to certain subgroups of the displacement group for
, which are isomorphic to certain subgroups of the displacement group for 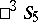 . There is also a relation between the groups of motions for these three-dimensional spaces (elliptic and hyperbolic) and groups of displacements of spaces of lower dimensions (see Fubini model; Kotel'nikov interpretation). The Plücker interpretation is also used in examining the interpretation of the three-dimensional symplectic space
. There is also a relation between the groups of motions for these three-dimensional spaces (elliptic and hyperbolic) and groups of displacements of spaces of lower dimensions (see Fubini model; Kotel'nikov interpretation). The Plücker interpretation is also used in examining the interpretation of the three-dimensional symplectic space  in
in  .
.
The Plücker interpretation was proposed by J. Plücker [1].
References
| [1] | J. Plücker, "Neue Geometrie des Raumes gegründet auf die Betrachtung der geraden Linie als Raumelement" , Teubner (1868) |
| [2] | B.A. Rozenfel'd, "Non-Euclidean geometry" , Moscow (1955) (In Russian) |
| [3] | F. Klein, "Vorlesungen über höhere Geometrie" , Springer (1926) |
Comments
The quadric in 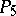 whose points represent the lines in
whose points represent the lines in 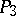 is often referred to as the Plücker quadric.
is often referred to as the Plücker quadric.
References
| [a1] | J.W.P. Hirschfeld, "Finite projective spaces of three dimensions" , Oxford Univ. Press (1985) |
| [a2] | B.L. van der Waerden, "Einführung in die algebraische Geometrie" , Springer (1939) pp. Chapt. 1 |
| [a3] | D.M.Y. Sommerville, "Analytical geometry of three dimensions" , Cambridge Univ. Press (1934) |
Plücker interpretation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Pl%C3%BCcker_interpretation&oldid=12938