Second boundary value problem
From Encyclopedia of Mathematics
One of the boundary value problems (cf. Boundary value problem, partial differential equations) for partial differential equations. For example, let there be given a second-order elliptic equation
 | (*) |
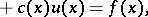 |
where 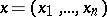 ,
,  , in a bounded domain
, in a bounded domain  , with a normal at each point of the boundary
, with a normal at each point of the boundary 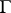 . The second boundary value problem for equation (*) in
. The second boundary value problem for equation (*) in  is the following problem: Out of the set of all solutions of equation (*), isolate those solutions which have, at all boundary points, derivatives with respect to the interior normal
is the following problem: Out of the set of all solutions of equation (*), isolate those solutions which have, at all boundary points, derivatives with respect to the interior normal 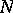 and which satisfy the condition
and which satisfy the condition
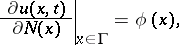 |
where 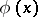 is a given function. The second boundary value problem is also known as the Neumann problem.
is a given function. The second boundary value problem is also known as the Neumann problem.
References
| [1] | A.V. Bitsadze, "Boundary value problems for second-order elliptic equations" , North-Holland (1968) (Translated from Russian) |
| [2] | V.S. Vladimirov, "Equations of mathematical physics" , MIR (1984) (Translated from Russian) |
| [3] | C. Miranda, "Partial differential equations of elliptic type" , Springer (1970) (Translated from Italian) |
| [4] | I.G. Petrovskii, "Partial differential equations" , Saunders (1967) (Translated from Russian) |
Comments
References
| [a1] | P.R. Garabedian, "Partial differential equations" , Wiley (1963) |
| [a2] | R. Courant, D. Hilbert, "Methods of mathematical physics. Partial differential equations" , 2 , Interscience (1965) (Translated from German) |
How to Cite This Entry:
Second boundary value problem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Second_boundary_value_problem&oldid=12888
Second boundary value problem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Second_boundary_value_problem&oldid=12888
This article was adapted from an original article by A.K. Gushchin (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article