Recursive sequence
From Encyclopedia of Mathematics
recurrent sequence
A sequence 
that satisfies a relation
 |
where 
are constants. The relation permits one to compute the terms of the sequence one by one, in succession, if the first 
terms are known. A classical example of such a sequence is the Fibonacci sequence 
(
, 
). A recursive series is a power series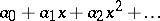 
whose coefficients form a recursive sequence. Such a series represents an everywhere-defined rational function.
Comments
A good reference treating many aspects of such sequences is [a1].
References
| [a1] | A.J. van der Poorten, "Some facts that should be better known, especially about rational functions" R.A. Molin (ed.) , Number theory and applications (Proc. First Conf. Canadian Number Theory Assoc., Banff, April 1988) , Kluwer (1989) pp. 497–528 |
How to Cite This Entry:
Recursive sequence. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Recursive_sequence&oldid=12807
Recursive sequence. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Recursive_sequence&oldid=12807
This article was adapted from an original article by BSE-3 (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article