Lah number

A coefficient in the expansion

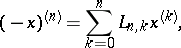where

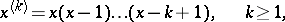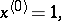are the falling factorials.

Replacing by 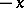, it follows that

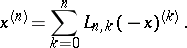The Lah numbers are given explicitly by

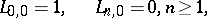and they are tabulated in [a1] for .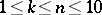

The numbers satisfy the recurrence relation

and have the generating function

They are related to Stirling numbers of the first and second kinds (cf. Combinatorial analysis), and to Bell polynomials (cf. Bell polynomial) by

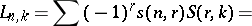See also [a4] for a connection with Laguerre polynomials.

If 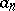and 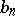are sequences, then

References

[a1]	L. Comtet, "Advanced combinatorics" , Reidel (1974)
[a2]	I. Lah, "Eine neue Art von Zahlen, ihre Eigenschaften und Anwendung in der mathematischen Statistik" Mitteil. Math. Statist. , 7 (1955) pp. 203–216
[a3]	J. Riordan, "Combinatorial analysis" , Wiley (1958)
[a4]	S. Roman, "The umbral calculus" , Acad. Press (1984)