Nil ideal
From Encyclopedia of Mathematics
A subset 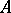 of a ring
of a ring 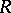 is called nil if each element of it is nilpotent (cf. Nilpotent element). An ideal of
is called nil if each element of it is nilpotent (cf. Nilpotent element). An ideal of 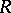 is a nil ideal if it is a nil subset. There is a largest nil ideal, which is called the nil radical. One has that
is a nil ideal if it is a nil subset. There is a largest nil ideal, which is called the nil radical. One has that
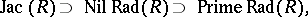 |
where 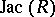 denotes the Jacobson radical of
denotes the Jacobson radical of  and
and  is the prime radical of
is the prime radical of 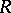 , i.e. the intersection of all prime ideals of
, i.e. the intersection of all prime ideals of  . Each of the inclusions can be proper. If
. Each of the inclusions can be proper. If 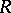 is commutative,
is commutative, 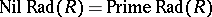 . The prime radical is also called the lower nil radical, and the nil radical the upper nil radical.
. The prime radical is also called the lower nil radical, and the nil radical the upper nil radical.
References
| [a1] | C. Faith, "Algebra" , II. Ring theory , Springer (1976) |
| [a2] | J.C. McConnell, J.C. Robson, "Noncommutative Noetherian rings" , Wiley (1987) |
| [a3] | L.H. Rowen, "Ring theory" , 1 , Acad. Press (1988) |
How to Cite This Entry:
Nil ideal. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Nil_ideal&oldid=12426
Nil ideal. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Nil_ideal&oldid=12426