Weak relative minimum

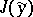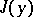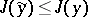A minimal value attained by a functional on a curve , , such that for all comparison curves 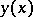satisfying the first-order -proximity condition

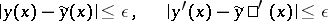(1)

throughout the interval 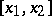. It is assumed that the curves 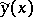, 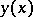satisfy the given boundary conditions.

If in (1) one disregards the -proximity condition on the derivative, then this leads to the zero-order -proximity condition. The minimal value of the functional 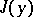in a zero-order -neighbourhood is called a strong relative minimum.

Since the zero-order -proximity condition distinguishes a wider class of curves than the first-order -proximity condition, every strong relative minimum is also a weak relative minimum, but not conversely.

For an extremal 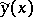to give a weak relative minimum, the Legendre condition must hold on it. For a strong relative minimum, the more general Weierstrass conditions (for a variational extremum) must hold. In terms of optimal control theory, the distinction between these necessary conditions means that for a weak (strong) relative minimum, it is necessary that, at the points of the extremal, the Hamilton function have a local maximum (absolute maximum) with respect to the control (in agreement with the Pontryagin maximum principle).

Sufficient conditions for a weak relative minimum impose certain requirements only on the extremal , whereas in the case of a strong minimum, one requires conditions similar in the sense to hold not only on 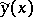, but also in a certain zero-order -neighbourhood of it. An extremal will be a weak relative minimum if the strong Legendre and strong Jacobi conditions hold along it. An extremal will be a strong relative minimum if it can be imbedded in a field of extremals at all points of which the Weierstrass function is non-negative.

References

[1]	M.A. Lavrent'ev, L.A. Lyusternik, "A course in variational calculus" , Moscow-Leningrad (1950) (In Russian)
[2]	V.I. Smirnov, "A course of higher mathematics" , 4 , Addison-Wesley (1964) (Translated from Russian)

References

[a1]	D.G. Luenberger, "Optimization by vector space methods" , Wiley (1969)
[a2]	L. Cesari, "Optimization - Theory and applications" , Springer (1983)