Laguerre formula

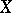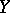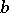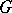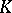A formula for calculating the angle between straight lines in Euclidean and pseudo-Euclidean spaces. Let and be the points at infinity on two straight lines and and let and be the points of intersection of these lines with the absolute of the space. Then the angle between these lines can be expressed in terms of the cross ratio :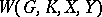

For two-dimensional pseudo-Euclidean space, and are the direction vectors of the isotropic lines passing through the point of intersection of the lines and .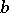

The formula was introduced by E. Laguerre .

A formula according to which, for all curves on a given surface that touch at some point, the quantity

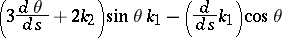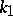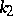is invariant, where and are the curvature and the torsion of the curve, is the angle between the principal normal of the curve and the normal to the surface, and is the natural parameter on the curve. The formula was obtained by E. Laguerre (1870, see [2]).

References

[1]	E. Laguerre, "Sur la théorie des foyers" Nouv. Ann. Math. , 12 (1853) pp. 57–66
[2]	E. Laguerre, "Oeuvres" , 2 , Chelsea, reprint (1972)
[3]	B.A. Rozenfel'd, "Non-Euclidean geometry" , Moscow (1955) (In Russian)

References

[a1]	M. Berger, "Geometry" , 1–2 , Springer (1987) (Translated from French)