Fractals

fractal sets

Originally defined by B.B. Mandelbrot as point sets with non-integer dimension in the sense of Hausdorff–Besicovitch (cf. Dimension). Classical examples are the triadic Cantor set and the non-differentiable curve obtained by von Koch. Typically, a fractal is self-similar in a deterministic or a stochastic way. D. Sullivan introduced the concept of quasi-self-similarity. A 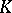-quasi-isometry is defined by a function 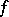acting on a metric space 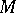with metric 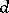satisfying

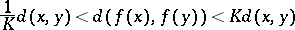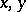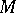for all in . A set 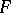is called quasi-self-similar if there exist a 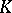and an 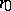such that multiplication by of 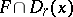maps into 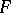by a quasi-isometry for all 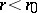and all 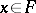. (Here is the open ball centred at of radius .) Accordingly, a fractal may be defined as a quasi-self-similar set. In some important cases the similarity transformations of a fractal set have the structure of a semi-group of non-expanding transformations with two or more generators. The Julia set of an analytic function 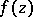is such a fractal, the inverses of 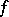being the generators of the corresponding semi-group. The fractal concept can be generalized in a variety of ways, but generally accepted definitions are still lacking. In one such a generalization the fractal dimension is only a local property. Multi-fractal measures are related to a distribution on a geometric support which could be a fractal set in the ordinary sense.

The field of fractals is rapidly expanding, in particular their applications in statistical physics, natural sciences and computer graphics. E.g., the use of fractals in image processing may give a considerable compression of relevant data.

Many "objects" in nature, such as, e.g., coastlines ([a1]), zeolites, patterns of dialectic discharge ([a5]), Anderson localized wave functions, dendritic growth and viscous fingers ([a6]), can be well described by deterministic or stochastic (multi-) fractal structures. Recently, [a5], progress has been made in understanding, in terms of Laplace equations and stochastic fields, how fractal structures could arise and evolve dynamically.

References

[a1]	B.B. Mandelbrot, "The fractal geometry of nature" , Freeman (1983)
[a2]	K.J. Falconer, "The geometry of fractal sets" , Cambridge Univ. Press (1985)
[a3]	H.-O. Peitgen, P.H. Richter, "The beauty of fractals" , Springer (1986)
[a4]	B.B. Mandelbrot, "Fractals and multifractals. Noise, turbulence and galaxies" , Springer (1988)
[a5]	L. Pietronero, C. Evertsz, A.P. Siebesma, "Fractal and multifractal structures in kinetic critical phenomena" S. Albeverio (ed.) Ph. Blanchard (ed.) M. Hazewinkel (ed.) L. Streit (ed.) , Stochastic processes in physics and engineering , Reidel (1988) pp. 253–278
[a6]	L. Pietronero (ed.) E. Tosatti (ed.) , Fractals in physics , North-Holland (1986)