Khinchin inequality

for independent functions

An estimate in 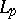of the sum of independent functions (cf. Independent functions, system of). Suppose that 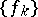is a system of independent functions and that for some ,

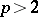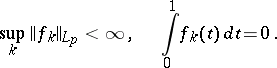Then

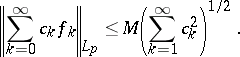If

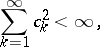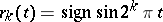is a Rademacher function (cf. Rademacher system) and if

then for any ,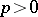

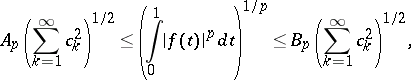where 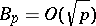as 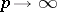. This inequality was established by A.Ya. Khinchin in [1]. The exact value of 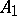is .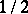

An analogue of the Khinchin inequality is valid in Banach spaces [4]. There exists a constant 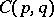, , 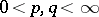such that for any element in a Banach space ,

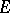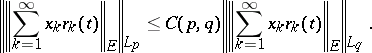One of the numerous applications of the Khinchin inequality is as follows: If

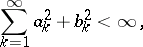then for almost-all choices 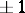the function

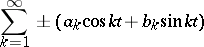belongs to all 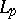, 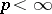(see [5]).

References

[1]	A.Ya. Khinchin, "Ueber dyadische Brüche" Math. Z. , 18 (1923) pp. 109–116
[2]	S. Karlin, "Orthogonal properties of independent functions" Trans. Amer. Math. Soc. , 66 (1949) pp. 44–64
[3]	V.F. Gaposhkin, "Lacunary series and independent functions" Russian Math. Surveys , 21 : 6 (1966) pp. 1–82 Uspekhi Mat. Nauk , 21 (1966) pp. 3–82
[4]	J.-P. Kahane, "Some random series of functions" , Cambridge Univ. Press (1985)
[5]	A. Zygmund, "Trigonometric series" , 1 , Cambridge Univ. Press (1988)