Pi(number)
The ratio of the length of a circle to its diameter; it is an infinite non-periodic decimal number
 |
One frequently arrives at the number  as the limit of certain arithmetic sequences involving simple laws. An example is Leibniz' series
as the limit of certain arithmetic sequences involving simple laws. An example is Leibniz' series
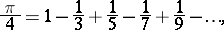 |
which, however, converges very slowly. There are more rapidly-converging series suitable for calculating  .
.
The possibility of a pure analytic definition of  is of essential significance for geometry. For example,
is of essential significance for geometry. For example,  also participates in certain formulas in non-Euclidean geometry, but not as the ratio of the length of a circle to its diameter (this ratio is not constant in non-Euclidean geometry). The arithmetic nature of
also participates in certain formulas in non-Euclidean geometry, but not as the ratio of the length of a circle to its diameter (this ratio is not constant in non-Euclidean geometry). The arithmetic nature of  was finally elucidated in analysis, with a decisive part played by Euler's formula:
was finally elucidated in analysis, with a decisive part played by Euler's formula:
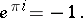 |
At the end of the 18th century, J. Lambert and A. Legendre established that 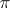 is an irrational number, while in the 19th century, F. Lindemann showed that
is an irrational number, while in the 19th century, F. Lindemann showed that 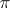 is a transcendental number.
is a transcendental number.
Comments
A nice account of Lindemann's proof can be found in [a3], Chapt. 6.
The number of known digits of  has increased exponentially in recent times. At the moment (1990), the record seems to be half a billion digits (D.V. Chudnovsky and G.V. Chudnovsky). For an account of such computations see [a1]. Up to the 1960's the standard way to calculate
has increased exponentially in recent times. At the moment (1990), the record seems to be half a billion digits (D.V. Chudnovsky and G.V. Chudnovsky). For an account of such computations see [a1]. Up to the 1960's the standard way to calculate  was to use Machin's formula
was to use Machin's formula 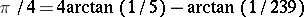 and the power series of
and the power series of  . Nowadays, some powerful formulas of Ramanujan are used. It is still not known how randomly the digits of
. Nowadays, some powerful formulas of Ramanujan are used. It is still not known how randomly the digits of 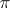 are distributed; in particular, whether
are distributed; in particular, whether  is a normal number.
is a normal number.
References
| [a1] | J.M. Borwein, P.B. Borwein, "Pi and the AGM" , Interscience (1987) |
| [a2] | P. Beckmann, "A history of pi" , The Golem Press , Boulder (Co.) (1971) |
| [a3] | I. Stewart, "Galois theory" , Chapman & Hall (1979) |
Pi(number). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Pi(number)&oldid=11364