Ellipsoidal harmonic

A function of a point on an ellipsoid that appears in the solution of the Laplace equation by the method of separation of variables in ellipsoidal coordinates.

Let be Cartesian coordinates in the Euclidean space , related to the ellipsoidal coordinates by three formulas of the same form

where , and . Putting , one obtains coordinate surfaces in the form of ellipsoids. A harmonic function that is a solution of the Laplace equation can be written as a linear combination of expressions of the form

(*)

where the factors , , 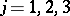are solutions of the Lamé equation. Expressions of the form (*) for and their linear combinations are called ellipsoidal harmonics or, better, surface ellipsoidal harmonics, in contrast to combinations of expressions (*) depending on all three variables , which are sometimes called spatial ellipsoidal harmonics.

References

[1]	A.N. [A.N. Tikhonov] Tichonoff, A.A. Samarskii, "Differentialgleichungen der mathematischen Physik" , Deutsch. Verlag Wissenschaft. (1959) (Translated from Russian)
[2]	P.M. Morse, H. Feshbach, "Methods of theoretical physics" , 1–2 , McGraw-Hill (1953)