Namioka theorem

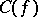Let be a regular, strongly countably complete topological space (cf. also Strongly countably complete topological space), let be a locally compact and -compact space (cf. also Compact space) and let be a pseudo-metric space. In 1974, I. Namioka [a7] proved that for every separately continuous function there is a dense -subset of such that the set is contained in , the set of points of continuity of 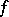(cf. also Set of type (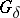); Separate and joint continuity).

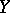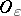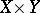The original proof of this theorem starts with an interesting reduction to the case when is compact. Next, using purely topological methods, such as, e.g., the Arkhangel'skii–Frolík covering theorem and Kuratowski's theorem on closed projections, Namioka shows that, given that the set is the union of all open subsets of such that 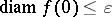, the set is dense in .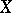

For 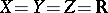(the real numbers), such a result was known already to R. Baire [a2] (cf. Separate and joint continuity).

If 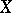is complete metric, is compact metric and 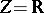, Namioka's theorem was shown by H. Hahn [a6] (see also [a11]).

The question whether the completeness of 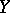suffices in Hahn's result was asked, independently, in [a1] and [a5]. The following example, due to J.B. Brown [a8] shows that completeness does not suffice and proves the necessity of compactness of . In fact, let , , where 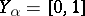and 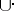denotes the free union of, in fact, many copies of . Let 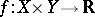be separately continuous on every "square" and having a point of discontinuity along the line 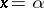. Then, clearly, the set 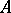mentioned in Namioka's theorem is empty.

Answering a problem of Namioka, it was shown [a12] that Namioka's theorem fails for all Baire spaces 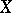(cf. also Baire space). Still, the theorem holds for certain Banach–Mazur game-defined spaces (cf. also Banach–Mazur game), namely for 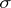--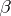defavourable spaces [a3], [a10] and for Baire spaces having dense subsets that are countable unions of 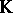-analytic subsets [a13].

The importance of Namioka's theorem lies in the fact that both 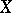and 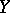are neither metrizable nor having any kind of countability of basis.

If has a countable base, then Namioka's theorem holds for all Baire spaces , see [a4] and [a9].

For further information, see Namioka space.

References

[a1]	A. Alexiewicz, W. Orlicz, "Sur la continuité et la classification de Baire des fonctions abstraites" Fundam. Math. , 35 (1948) pp. 105–126
[a2]	R. Baire, "Sur les fonctions des variables réelles" Ann. Mat. Pura Appl. , 3 (1899) pp. 1–122
[a3]	A. Bouziad, "Jeux topologiques et point de continuité d'une application séparément continue" C.R. Acad. Sci. Paris , 310 (1990) pp. 359–361
[a4]	J. Calbrix, J.P. Troallic, "Applications séparément continue" C.R. Acad. Sci. Paris Sér. A , 288 (1979) pp. 647–648
[a5]	J.P.R. Christensen, "Joint continuity of separately continuous functions" Proc. Amer. Math. Soc. , 82 (1981) pp. 455–461
[a6]	H. Hahn, "Reelle Funktionen" , Leipzig (1932) pp. 325–338
[a7]	I. Namioka, "Separate and joint continuity" Pacific J. Math. , 51 (1974) pp. 515–531
[a8]	Z. Piotrowski, "Separate and joint continuity" Real Analysis Exchange , 11 (1985/86) pp. 293–322
[a9]	Z. Piotrowski, "Topics in separate and joint continuity" in preparation (2001)
[a10]	J. Saint-Raymond, "Jeux topologiques et espaces de Namioka" Proc. Amer. Math. Soc. , 87 (1983) pp. 499–504
[a11]	R. Sikorski, "Funkcje rzeczywiste" , I , PWN (1958) pp. 172; Problem () (In Polish)
[a12]	M. Talagrand, "Propriété de Baire et propriété de Namioka" Math. Ann. , 270 (1985) pp. 159–174
[a13]	G. Debs, "Points de continuité d'une fonction séparément continue" Proc. Amer. Math. Soc. , 97 (1986) pp. 167–176