Busemann function

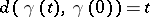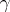A concept of function which measures the distance to a point at infinity. Let be a Riemannian manifold. The Riemannian metric induces a distance function on . Let be a ray in , i.e., a unit-speed geodesic line such that for all . The Busemann function with respect to is defined by

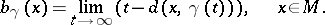Since is bounded above by and is monotone non-decreasing in , the limit always exits. It follows that 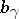is a Lipschitz function with Lipschitz constant 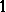. The level surfaces 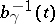of a Busemann function are called horospheres. Busemann functions can also be defined on intrinsic (or length) metric spaces, in the same manner. Actually, H. Busemann [a2] first introduced them on so-called 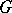-spaces and used them to state the parallel axiom on straight 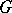-spaces (cf. also Closed geodesic).

If has non-negative sectional curvature, 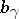is convex, see [a3]. If 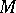has non-negative Ricci curvature, 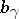is a subharmonic function, see [a4]. If 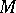is a Kähler manifold with non-negative holomorphic bisectional curvature, 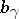is a plurisubharmonic function, see [a7]. If 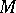is a Hadamard manifold, 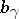is a 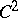concave function, see [a9], [a2], and, moreover, the horospheres are 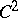-hypersurfaces, see [a9]. On the Poincaré model of the hyperbolic space, the horospheres coincide with the Euclidean spheres in 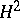which are tangent to the sphere at infinity. On Hadamard manifolds, it is more customary to call 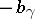the Busemann function instead of .

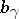More recently, M. Gromov [a1] introduced a generalization of the concept of Busemann function called the horofunction. Let 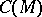be the set of continuous functions on 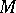and let 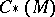the quotient space of 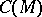modulo the constant functions. Use the topology on 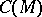induced from the uniform convergence on compact sets and its quotient topology on 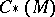. The embedding of 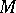into defined by 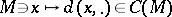induces an embedding 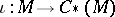. The closure of the image 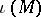is a compactification of (cf. also Compactification). 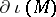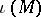According to [a1], [a8], a horofunction is defined to be a class (or an element of a class) in the topological boundary of in 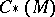. Any Busemann function is a horofunction. For Hadamard manifolds, any horofunction can be represented as some Busemann function, see [a1]. However, this is not necessarily true for non-Hadamard manifolds. Horofunctions have been defined not only for Riemannian manifolds but also for complete locally compact metric spaces.

Let 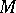be a complete non-compact Riemannian manifold with non-negative sectional curvature, and for , let 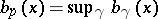, , where 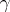runs over all rays emanating from 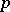. Then, 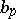is a convex exhaustion function, see [a3], that is, a functi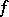on on 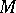such that 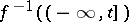is compact for any 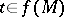. The function 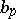plays an important role in the first step of the Cheeger–Gromoll structure theory for 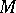, see [a3]. Any Kähler manifold admitting a strictly plurisubharmonic exhaustion function is a Stein manifold, see [a5]. This, together with the use of Busemann functions or 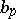, yields various sufficient conditions for a Kähler manifold to be Stein; see, for example, [a7], [a17]. Some results for the exhaustion property of Busemann functions are known, see [a14], [a15], [a12], [a13]. For a generalization of the notion of a horofunction and of 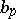, see [a18]. A general reference for Busemann function and its related topics is [a16].

References

[a1]	W. Ballmann, M. Gromov, V. Schroeder, "Manifolds of nonpositive curvature" , Progr. Math. , 61 , Birkhäuser (1985)
[a2]	H. Busemann, "The geometry of geodesics" , Acad. Press (1955)
[a3]	J. Cheeger, D. Gromoll, "On the structure of complete manifolds of nonnegative curvature" Ann. of Math. (2) , 96 (1972) pp. 413–443
[a4]	J. Cheeger, D. Gromoll, "The splitting theorem for manifolds of nonnegative Ricci curvature" J. Diff. Geom. , 6 (1971/72) pp. 119–128
[a5]	F. Docquier, H. Grauert, "Leisches Problem und Rungescher Satz für Teilgebiete Steinscher Mannigfaltigkeiten" Math. Ann. , 140 (1960) pp. 94–123
[a6]	P. Eberlein, B. O'Neill, "Visibility manifolds" Pacific J. Math. , 46 (1973) pp. 45–109
[a7]	R.E. Greene, H. Wu, "On Kähler manifolds of positive bisectional curvature and a theorem of Hartogs" Abh. Math. Sem. Univ. Hamburg , 47 (1978) pp. 171–185 (Special issue dedicated to the seventieth birthday of Erich Käler)
[a8]	M. Gromov, "Structures métriques pour les variétés riemanniennes" , Textes Mathématiques [Mathematical Texts] , 1 , CEDIC (1981) (Edited by J. Lafontaine and P. Pansu)
[a9]	E. Heintze, H.-C. Im Hof, "Geometry of horospheres" J. Diff. Geom. , 12 : 4 (1977) pp. 481–491 (1978)
[a10]	N. Innami, "On the terminal points of co-rays and rays" Arch. Math. (Basel) , 45 : 5 (1985) pp. 468–470
[a11]	N. Innami, "Differentiability of Busemann functions and total excess" Math. Z. , 180 : 2 (1982) pp. 235–247
[a12]	A. Kasue, "A compactification of a manifold with asymptotically nonnegative curvature" Ann. Sci. Ecole Norm. Sup. 4 , 21 : 4 (1988) pp. 593–622
[a13]	Z. Shen, "On complete manifolds of nonnegative 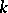th-Ricci curvature" Trans. Amer. Math. Soc. , 338 : 1 (1993) pp. 289–310
[a14]	K. Shiohama, "Busemann functions and total curvature" Invent. Math. , 53 : 3 (1979) pp. 281–297
[a15]	K. Shiohama, "The role of total curvature on complete noncompact Riemannian -manifolds" Illinois J. Math. , 28 : 4 (1984) pp. 597–620
[a16]	K. Shiohama, "Topology of complete noncompact manifolds" , Geometry of Geodesics and Related Topics (Tokyo, 1982) , Adv. Stud. Pure Math. , 3 , North-Holland (1984) pp. 423–450
[a17]	Y.T. Siu, S.T. Yau, "Complete Kähler manifolds with nonpositive curvature of faster than quadratic decay" Ann. of Math. (2) , 105 : 2 (1977) pp. 225–264
[a18]	H. Wu, "An elementary method in the study of nonnegative curvature" Acta Math. , 142 : 1–2 (1979) pp. 57–78