Lannes-T-functor
The calculation of the homotopy type of the space of continuous mappings 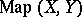 is a fundamental problem of homotopy theory. The set of path components,
is a fundamental problem of homotopy theory. The set of path components, 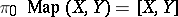 corresponds to the homotopy classes of such mappings. There are relatively few cases for which this information is explicitly known (as of 1998). A major impact of the work [a1] of J. Lannes on unstable modules and the T-functor has been to expand this knowledge to include many cases in which the sources and targets are classifying spaces of finite and compact Lie groups (cf. also Lie group).
corresponds to the homotopy classes of such mappings. There are relatively few cases for which this information is explicitly known (as of 1998). A major impact of the work [a1] of J. Lannes on unstable modules and the T-functor has been to expand this knowledge to include many cases in which the sources and targets are classifying spaces of finite and compact Lie groups (cf. also Lie group).
The work of N. Steenrod and others assigns in a natural way to each topological space 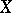 and each prime number
and each prime number  an algebraic model, consisting of a graded algebra
an algebraic model, consisting of a graded algebra 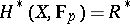 over
over  and an algebra
and an algebra 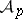 of natural operations, called the Steenrod algebra. Each
of natural operations, called the Steenrod algebra. Each 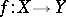 induces an element
induces an element 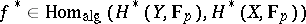 that commutes with the action of
that commutes with the action of 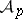 .
.  is a connected graded Hopf algebra acting on the graded algebra
is a connected graded Hopf algebra acting on the graded algebra 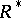 .
.
The hypothesis that 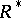 is the cohomology of a space imposes an additional "unstable" condition. This is most simply stated if
is the cohomology of a space imposes an additional "unstable" condition. This is most simply stated if 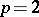 :
:  is generated as an (non-commutative) algebra by the Steenrod operations
is generated as an (non-commutative) algebra by the Steenrod operations  , with relations forced by its actions of the cohomology of all topological spaces. For example,
, with relations forced by its actions of the cohomology of all topological spaces. For example, 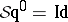 and
and 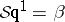 , the modulo-
, the modulo- Bockstein operator. The unstable condition is then that
Bockstein operator. The unstable condition is then that  for
for 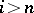 and
and  . The algebraic category
. The algebraic category  of unstable algebras
of unstable algebras 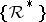 over
over 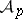 is thus an approximation to the homotopy category of topological spaces. The larger category
is thus an approximation to the homotopy category of topological spaces. The larger category 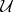 of unstable modules over
of unstable modules over 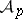 has also proved useful.
has also proved useful.
For 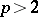 , the structure of
, the structure of 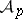 and unstable actions are similar, but slightly more involved. However, in all cases, the set of relations in the Steenrod algebra and the unstable condition are derivable from the known action of
and unstable actions are similar, but slightly more involved. However, in all cases, the set of relations in the Steenrod algebra and the unstable condition are derivable from the known action of 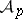 on the cohomology of products of copies of
on the cohomology of products of copies of  . In the following, explicit references to the coefficients are omitted.
. In the following, explicit references to the coefficients are omitted.
The relationship of 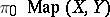 to its model
to its model 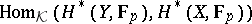 is of particular interest. The equivalence
is of particular interest. The equivalence
 |
raises the hope that in very favourable cases the mapping
 |
 |
might be an isomorphism. That suggests that in the category 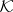 ,
, 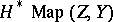 should be approximated by the left adjoint functor to tensoring on the right by
should be approximated by the left adjoint functor to tensoring on the right by 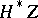 . This motivated J. Lannes to define the functor
. This motivated J. Lannes to define the functor 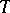 as follows: If
as follows: If 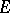 is a finite-dimensional
is a finite-dimensional 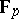 -vector space, then the
-vector space, then the  -functor
-functor 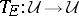 is the left adjoint in
is the left adjoint in 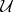 of the functor
of the functor 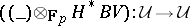 . In the topological case, there is a natural mapping
. In the topological case, there is a natural mapping
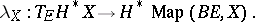 |
For general 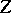 , the adjoint to
, the adjoint to 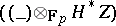 accounts for only part of the starting page of a Bousfield–Kan unstable Adams spectral sequence for
accounts for only part of the starting page of a Bousfield–Kan unstable Adams spectral sequence for 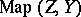 . Lannes provides the basic connection to topology by blending the algebraic properties of
. Lannes provides the basic connection to topology by blending the algebraic properties of 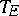 and
and 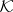 with the Bousfield–Kan spectral sequence: For many interesting spaces
with the Bousfield–Kan spectral sequence: For many interesting spaces 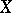 ,
,
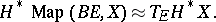 |
In particular,
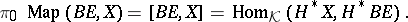 |
For 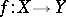 , one has the path component
, one has the path component 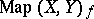 of functions homotopic to
of functions homotopic to 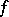 . The analogous
. The analogous 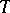 -construct is as follows: Each
-construct is as follows: Each 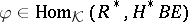 induces a
induces a  -module structure on
-module structure on 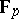 and
and
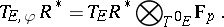 |
The most striking features of  are summarized below (see also [a1]). To some extent, these were presaged by work of G. Carlsson and H.T. Miller, who established that the
are summarized below (see also [a1]). To some extent, these were presaged by work of G. Carlsson and H.T. Miller, who established that the 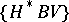 are injectives in
are injectives in 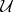 .
.
a) 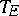 is exact.
is exact.
b) 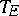 respects tensor products, i.e
respects tensor products, i.e 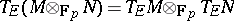 .
.
c)  commutes with the
commutes with the 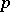 th power operations in a suitable sense.
th power operations in a suitable sense.
d)  maps
maps 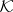 to
to 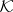 .
.
In principle, 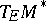 can be calculated by using the exactness property and a resolution of
can be calculated by using the exactness property and a resolution of 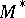 by free unstable
by free unstable 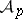 -modules. In practice, other methods are often more effective; for example,
-modules. In practice, other methods are often more effective; for example,
1) If 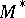 is finite, then
is finite, then 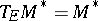 .
.
2) If 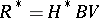 , then
, then
 |
for 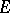 and
and 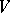 finite-dimensional
finite-dimensional 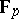 -vector spaces.
-vector spaces.
3) If 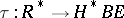 in
in 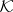 is an inclusion, then
is an inclusion, then 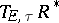 is the smallest sub-Hopf algebra of
is the smallest sub-Hopf algebra of 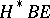 that contains
that contains 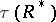 .
.
4) If 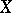 is a finite
is a finite 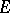 -complex with fixed point set
-complex with fixed point set 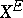 and
and 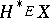 is the modulo
is the modulo  cohomology of the Borel construction, then
cohomology of the Borel construction, then 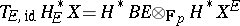 in
in  .
.
5) If 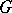 is a compact Lie group, then
is a compact Lie group, then
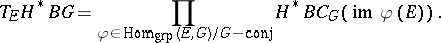 |
These examples each have powerful topological consequences. For example, the first and fourth lead to new proofs of the Sullivan conjecture, originally proved by Miller and Carlsson. The last leads to a new view of the homotopy theory of classifying spaces. Most of the above is referenced in [a2].
References
| [a1] | J. Lannes, "Sur les espaces fonctionnels dont la source est le classifiant d'un  -groupe abélien élémentaire" Inst. Hautes Etudes Sci. Publ. Math. , 75 (1992) pp. 135–244 (Appendix by M. Zisman) -groupe abélien élémentaire" Inst. Hautes Etudes Sci. Publ. Math. , 75 (1992) pp. 135–244 (Appendix by M. Zisman) |
| [a2] | L. Schwartz, "Unstable modules over the Steenrod algebra and Sullivan's fixed point set conjecture" , Univ. Chicago Press (1994) |
Lannes-T-functor. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lannes-T-functor&oldid=50376