Artin root numbers

A global Artin root number is a complex number 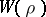of modulus 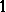appearing in the functional equation of an Artin 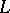-series (cf. also 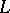-function)

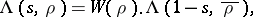(a1)

in which is a representation

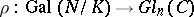of the Galois group of a finite Galois extension 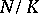of global fields (cf. also Representation theory; Galois theory; Extension of a field), denotes the complex-conjugate representation, and 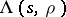is the (extended) Artin 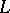-series with gamma factors at the Archimedean places of 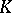(details can be found in [a6]).

Work of R. Langlands (unpublished) and P. Deligne [a2] shows that the global Artin root number can be written canonically as a product

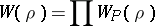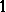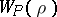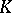of other complex numbers of modulus , called local Artin root numbers (Deligne calls them simply "local constants" ). Given , there is one local root number for each non-trivial place of the base field , and 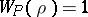for almost all .

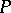Interest in root numbers arises in part because they are analogues of Langlands' -factors appearing in the functional equations of 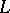-series associated to automorphic forms. In special settings, global root numbers are known to have deep connections to the vanishing of Dedekind zeta-functions at 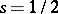(cf. also Dedekind zeta-function), and to the existence of a global normal integral basis, while local root numbers are connected to Stiefel–Whitney classes, to Hasse symbols of trace forms, and to the existence of a canonical quadratic refinement of the local Hilbert symbol. Excellent references containing both a general account as well as details can be found in [a6], [a11] and [a4].

Some observations.

a) The global and the local root numbers of 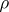depend only on the isomorphism class of 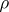; hence the root numbers are functions of the character .

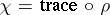b) When the character of 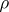is real-valued, then the global root number 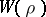has value 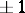, and each local root number is a fourth root of unity.

c) [a1] When 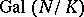has a representation 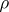whose character is real-valued and whose global root number 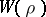is 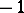, then the Dedekind zeta-function 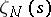vanishes at .

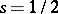d) [a5] When 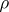is a real representation (a condition stronger than the requirement that the character be real-valued), then the global root number 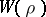is 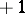. This means that the product of the local root numbers of a real representation is 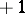, so the Fröhlich–Queyrut theorem is a reciprocity law (cf. Reciprocity laws), or a "product formula" , for local root numbers. Some authors write "real orthogonal" or just "orthogonal" in place of "real representation" ; all three concepts are equivalent.

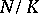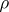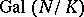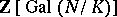e) [a12] A normal extension of number fields has a normal integral basis if and only if is at most tamely ramified and the global root number for all irreducible symplectic representations of . (By definition, the extension has a normal integral basis provided the ring of integers is a free -module).

f) [a3] Let 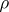be a real representation and let 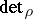be the 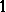-dimensional real representation obtained by composing 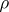with the determinant. Then for each place 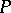of , the normalized local Artin root number 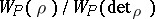equals the second Stiefel–Whitney class 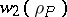of the restriction of 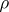to a decomposition subgroup of in .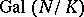

g) [a9] Let 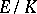be a finite extension of number fields, with normal closure 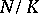. Let 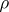be the representation of 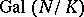induced by the trivial representation of . Then the Hasse symbol at 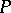of the trace form is given by , where is the discriminant of the trace form and 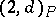is a Hilbert symbol (cf. Norm-residue symbol).

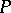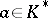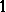h) [a11] For a place and a non-zero element , the -dimensional real representation 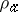sending 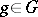to 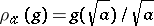has a local root number 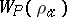, which will be abbreviated by . For 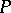fixed, these local root numbers produce a mapping

which satisfies

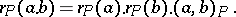(a2)

The last factor is the local Hilbert symbol at ; it gives a non-degenerate inner product on the local square class group at 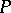, viewed as a vector space over the field of two elements, the latter identified with 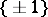. Equation (a2) has been interpreted in [a7] to mean that the local root numbers give a canonical "quadratic refinement" of this inner product.

Remarks.

1) It follows formally from (a1) that 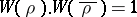. Moreover, , so the global root number 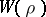has modulus 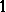. When the character of is real-valued, then 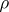and 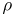are isomorphic, so their global root numbers are equal: . It follows that the global root number .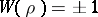

2) Statement c) follows from the basic argument in [a1], Sect. 3, with minor modifications.

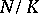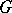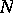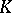3) To put Taylor's theorem in context, let be a finite Galois extension of fields, with Galois group . Then the normal basis theorem of field theory says that has a -basis consisting of the Galois conjugates of a single element; restated, 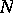is a free 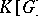-module. When 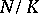is an extension of number fields, one can ask for a normal integral basis. There are two different notions: One can require the ring of integers 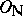to be a free 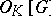module (necessarily of rank 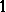), or one can require 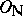to be a free 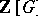-module (necessarily of rank 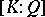). These notions coincide when the base field 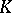is the field of rational numbers. At present (1998), little is known about the first notion, so the second is chosen. 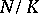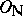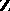Thus, has a normal integral basis when has a -basis 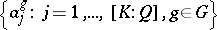. By results of E. Noether and R. Swan (see [a4], pp. 26–28), a necessary condition for 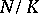to have a normal integral basis is that 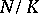be at most tamely ramified. A. Fröhlich conjectured and M. Taylor proved that the extra conditions beyond tameness needed to make 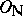a free -module is for all the global root numbers of symplectic representations to have value .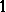

To say that a complex representation is symplectic means that the representation has even dimension, 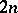, and factors through the symplectic group . The character values of a symplectic representation are real. A useful criterion is: When 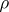is irreducible with character 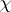, then the sum

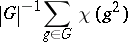takes the value 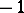when 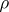is symplectic, the value 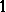when 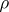is real, and the value in all other cases (see [a10], Prop. 39).

4) The families of complex numbers which can be realized as the local root numbers of some real representation of the Galois group of some normal extension 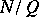have been determined in [a8].

References

[a1]	J.V. Armitage, "Zeta functions with zero at 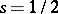" Invent. Math. , 15 (1972) pp. 199–205
[a2]	P. Deligne, "Les constantes des équation fonctionelles des fonctions 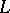" , Lecture Notes Math. , 349 , Springer (1974) pp. 501–597
[a3]	P. Deligne, "Les constantes locales de l'équation fonctionelle des fonction d'Artin d'une répresentation orthogonale" Invent. Math. , 35 (1976) pp. 299–316
[a4]	A. Fröhlich, "Galois module structure of algebraic integers" , Ergebn. Math. , 1 , Springer (1983)
[a5]	A. Fröhlich, J. Queyrut, "On the functional equation of the Artin L-function for characters of real representations" Invent. Math. , 20 (1973) pp. 125–138
[a6]	J. Martinet, "Character theory and Artin L-functions" , Algebraic Number Fields: Proc. Durham Symp. 1975 , Acad. Press (1977) pp. 1–87
[a7]	R. Perlis, "Arf equivalence I" , Number Theory in Progress: Proc. Internat. Conf. in Honor of A. Schinzel (Zakopane, Poland, June 30--July 9, 1997) , W. de Gruyter (1999)
[a8]	R. Perlis, "On the analytic determination of the trace form" Canad. Math. Bull. , 28 : 4 (1985) pp. 422–430
[a9]	J-P. Serre, "L'invariant de Witt de la forme " Comment. Math. Helvetici , 59 (1984) pp. 651–676
[a10]	J-P. Serre, "Représentations linéaires des groupes finis" , Hermann (1971) (Edition: Second)
[a11]	J. Tate, "Local constants" , Algebraic Number Fields: Proc. Durham Symp. 1975 , Acad. Press (1977) pp. 89–131
[a12]	M. Taylor, "On Fröhlich's conjecture for rings of integers of tame extensions" Invent. Math. , 63 (1981) pp. 41–79