Algebraic tangles
A family of tangles (cf. Tangle) defined recursively for any  as follows:
as follows:
i)  -algebraic tangles is the smallest family of
-algebraic tangles is the smallest family of  -tangles satisfying
-tangles satisfying
1) any  -tangle with
-tangle with  or
or 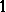 crossing is
crossing is  -algebraic;
-algebraic;
2) if 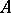 and
and 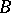 are
are  -algebraic tangles, then
-algebraic tangles, then  is
is  -algebraic for any integers
-algebraic for any integers  ,
,  , where
, where  denotes the rotation of a tangle by the angle
denotes the rotation of a tangle by the angle 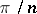 and
and  denotes (horizontal) composition of tangles.
denotes (horizontal) composition of tangles.
ii) If in condition 2) above, 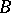 is restricted to tangles with no more than
is restricted to tangles with no more than 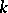 crossings, one obtains the family of
crossings, one obtains the family of  -algebraic tangles.
-algebraic tangles.
iii) If an 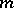 -tangle,
-tangle,  , is obtained from an
, is obtained from an 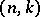 -algebraic tangle (respectively, an
-algebraic tangle (respectively, an  -algebraic tangle) by partially closing
-algebraic tangle) by partially closing 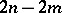 of its endpoints without a crossing, then
of its endpoints without a crossing, then 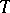 is called an
is called an 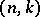 -algebraic
-algebraic 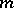 -tangle, respectively an
-tangle, respectively an  -algebraic
-algebraic  -tangle. For
-tangle. For  one obtains an
one obtains an  -algebraic link, respectively an
-algebraic link, respectively an  -algebraic link.
-algebraic link.
 -algebraic tangles were introduced by J.H. Conway (they are often called algebraic tangles in the sense of Conway or arborescent tangles). The
-algebraic tangles were introduced by J.H. Conway (they are often called algebraic tangles in the sense of Conway or arborescent tangles). The  -fold branched covering of
-fold branched covering of 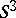 with a
with a  -algebraic link as a branched set is a Waldhausen graph manifold. Thus, not every link is
-algebraic link as a branched set is a Waldhausen graph manifold. Thus, not every link is  -algebraic. It is an open problem (as of 2001) to find, for a given
-algebraic. It is an open problem (as of 2001) to find, for a given  , a link which is not
, a link which is not  -algebraic. The smallest
-algebraic. The smallest  for which a link
for which a link 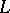 is
is  -algebraic is called the algebraic index of the link (it is bounded from above by the braid and bridge indices of the link). For example, the algebraic index of the
-algebraic is called the algebraic index of the link (it is bounded from above by the braid and bridge indices of the link). For example, the algebraic index of the 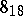 knot is equal to
knot is equal to  .
.
References
| [a1] | J.H. Conway, "An enumeration of knots and links" J. Leech (ed.) , Computational Problems in Abstract Algebra , Pergamon Press (1969) pp. 329–358 |
| [a2] | J.H. Przytycki, T. Tsukamoto, "The fourth skein module and the Montesinos–Nakanishi conjecture for  -algebraic links" J. Knot Th. Ramifications (2001) -algebraic links" J. Knot Th. Ramifications (2001) |
Algebraic tangles. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Algebraic_tangles&oldid=50242