Mehler-Fock-transform(2)
Mehler–Fok transform, Fock–Mehler transform, Fok–Mehler transform
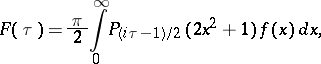 |
where  is the associated Legendre function of the first kind (cf. Legendre functions). This transform was introduced by F.G. Mehler [a1]. Some sufficient conditions for the inversion formula was found by V.A. Fock (also spelled V.A. Fok) [a2] and N.N. Lebedev [a3]. Some applications of the Mehler–Fock transform are given in [a7].
is the associated Legendre function of the first kind (cf. Legendre functions). This transform was introduced by F.G. Mehler [a1]. Some sufficient conditions for the inversion formula was found by V.A. Fock (also spelled V.A. Fok) [a2] and N.N. Lebedev [a3]. Some applications of the Mehler–Fock transform are given in [a7].
If  , then the integral
, then the integral  converges in the mean square with respect to the norm of the space
converges in the mean square with respect to the norm of the space  and is an isomorphism between these spaces. Moreover, the Parseval equality is true:
and is an isomorphism between these spaces. Moreover, the Parseval equality is true:
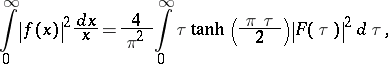 |
as well as the inversion formula
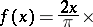 |
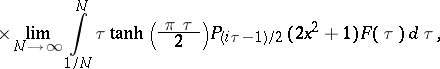 |
where the limit is taken with respect to the norm in  . As is shown, for instance, in [a5], the Mehler–Fock transform can be represented as the composition of the Hankel transform of index zero (cf. Integral transform; Hardy transform) and the Kontorovich–Lebedev transform.
. As is shown, for instance, in [a5], the Mehler–Fock transform can be represented as the composition of the Hankel transform of index zero (cf. Integral transform; Hardy transform) and the Kontorovich–Lebedev transform.
The generalized Mehler–Fock transform and its inverse involve the associated Legendre functions of the first kind 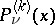 and are accordingly defined as:
and are accordingly defined as:
 |
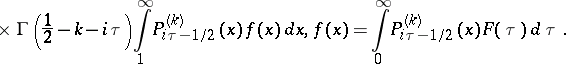 |
If  , these formulas reduce by simple substitutions to the ordinary Mehler–Fock transform. For
, these formulas reduce by simple substitutions to the ordinary Mehler–Fock transform. For 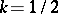 ,
, 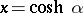 one obtains the Fourier cosine transform, while
one obtains the Fourier cosine transform, while 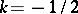 ,
,  leads to the Fourier sine transform.
leads to the Fourier sine transform.
If 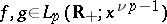 , where
, where 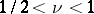 ,
,  , then for the Mehler–Fock transform of type (see [a5])
, then for the Mehler–Fock transform of type (see [a5])
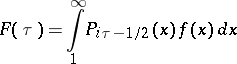 |
one can define the convolution operator (cf. also Convolution transform)
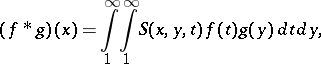 |
where 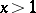 and
and
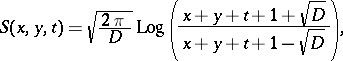 |
for 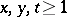 and
and 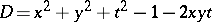 , where the main values of the square and the logarithm are taken (cf. also Logarithmic function).
, where the main values of the square and the logarithm are taken (cf. also Logarithmic function).
The convolution  belongs to the space
belongs to the space  and has the following representation:
and has the following representation:
 |
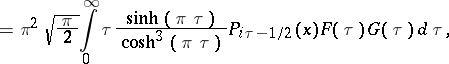 |
where 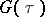 is the Mehler–Fock transform of the function
is the Mehler–Fock transform of the function  .
.
References
| [a1] | F.G. Mehler, "Ueber eine mit den Kugel- und cylinderfunctionen verwandte Function und ihre Anwendung in der Theorie der Electricitätsvertheilung" Math. Ann. , 18 (1881) pp. 161–194 |
| [a2] | V.A. Fock, "On the representation of an arbitrary function by integrals involving the Legendre function with a complex index" Dokl. Akad. Nauk SSSR , 39 : 7 (1943) pp. 279–283 (In Russian) |
| [a3] | N.N. Lebedev, "The Parseval theorem for the Mehler–Fock integral transform" Dokl. Akad. Nauk SSSR , 68 (1949) pp. 445–448 (In Russian) |
| [a4] | S.B. Yakubovich, "On the Mehler–Fock integral transform in 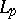 -spaces" Extracta Math. , 8 : 2–3 (1993) pp. 162–164 -spaces" Extracta Math. , 8 : 2–3 (1993) pp. 162–164 |
| [a5] | S.B. Yakubovich, "Index transforms" , World Sci. (1996) pp. Chap. 3 |
| [a6] | F. Oberhettinger, T.P. Higgins, "Tables of Lebedev, Mehler and generalized Mehler transforms" , Boeing Sci. Res. Lab. (1961) |
| [a7] | I.N. Sneddon, "The use of integral transforms" , McGraw-Hill (1972) pp. Chap. 7 |
Mehler-Fock-transform(2). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Mehler-Fock-transform(2)&oldid=50236