Wielandt subgroup

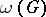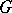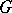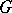The Wielandt subgroup of a group is defined to be the intersection of the normalizers of all the subnormal subgroups of (cf. also Subnormal subgroup; Normal subgroup; Normalizer of a subset). This characteristic subgroup was introduced in 1958 by H. Wielandt [a18]. Note that if and only if is a T-group, i.e. normality is transitive in .

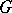When is nilpotent (cf. Nilpotent group), 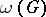is the intersection of the normalizers of all the subgroups of 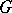. The latter is called the norm of and was introduced by R. Baer [a1]. In [a17], E. Schenkman showed that the norm is always contained in the second centre 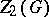(cf. also Centre of a group). Hence if 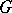is a nilpotent group.

The upper Wielandt series 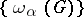of a group 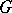is formed by iteration; thus,

where 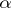is an ordinal and 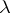a limit ordinal (cf. also Ordinal number). If 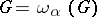for some ordinal 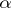, the smallest such 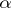is called the Wielandt length o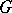f . If 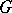has finite Wielandt length , then every subnormal subgroup of 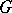has defect at most .

Wielandt [a18] proved that 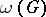contains all the subnormal (non-Abelian) simple subgroups of 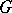, and also all the minimal normal subgroups of 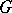that satisfy min-sn, the minimal condition on subnormal subgroups (cf. also Group with the minimum condition). This last result implies that 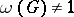whenever 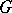is a non-trivial group with min-sn. Thus, the Wielandt subgroup is unexpectedly large for groups with min-sn.

A stronger result in this direction was found independently by D.J.S. Robinson [a15] and J.E. Roseblade [a16]: If 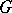is a group with min-sn, then 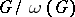is finite. Thus, a subnormal subgroup of 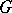has only finitely many conjugates. In addition, O.H. Kegel [a11] generalized the first of Wielandt's results by demonstrating that 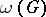contains all subnormal perfect T-subgroups of of 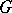. Since solvable T-groups are metAbelian ([a14]), 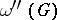is a perfect T-group. It follows that the join (cf. also Join) of all the subnormal perfect T-subgroups coincides with 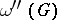in any group 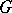. For a smooth treatment of these results see [a12].

The example of the infinite dihedral group shows that the Wielandt subgroup of a polycyclic group can easily be trivial. In 1991, J. Cossey [a7] showed that if 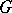is a polycyclic group, then 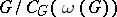is finite. Cossey also proved that 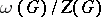is finite if 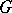is nilpotent-by-Abelian. Subsequently, this conclusion was extended to polycyclic groups that are meta-nilpotent or Abelian-by-finite by R. Brandl, S. Franciosi and F. de Giovanni. These authors were also able to show that if 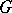is a finitely generated solvable-by-finite group of finite Prüfer rank (cf. Rank), then 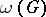is contained in the FC-centre (cf. also Group with a finiteness condition).

In some ways 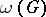might seem to be close to the centre for polycyclic groups 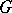. However, Cossey [a7] has pointed out that there is a nilpotent-by-finite polycyclic group 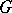such that 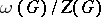is non-trivial and free Abelian. Cossey [a7] has also investigated the Wielandt length of a polycyclic group 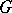, showing that this exists if and only if 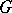is finite-by-nilpotent (when, of course, the Wielandt length is finite).

The Wielandt length of finite solvable groups has been investigated extensively by several authors, and its connection with other invariants such as the derived length and nilpotent length has been analyzed. Let 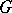be a finite solvable group with Wielandt length 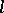. Since solvable T-groups are metAbelian, the derived length 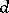of 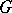is at most 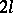. A.R. Camina [a5] improved this bound by showing that 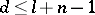, where is the nilpotent length of 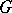and 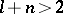. Camina also showed that 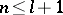. Subsequently, R. Bryce and J. Cossey [a4] gave the improved estimate , which is best possible when 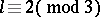. This work has been extended by C. Casolo [a6] to infinite solvable groups with finite Wielandt length. For more results on the Wielandt length of finite groups, see [a8].

In [a4], a local version of the Wielandt subgroup was introduced, 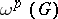where 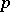is a prime number; this is the intersection of the normalizers of the 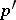-perfect subnormal subgroups, i.e. those that have no non-trivial -quotients. They showed that

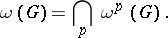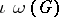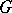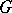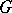Another variation of the Wielandt subgroup has been described in [a2]. The generalized Wielandt subgroup of a group is defined to be the intersection of the normalizers of all the infinite subnormal subgroups of . Thus, precisely when is an IT-group, i.e. all infinite subnormal subgroups of 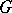are normal. The class of IT-groups has been investigated in [a10] and [a9]. J.C. Beidleman, M.R. Dixon and D.J.S. Robinson [a2] showed that the subgroups 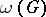and 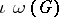are remarkably close, and indeed they coincide in many cases. In [a2] the following results were established. Let 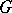be an infinite group; then:

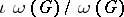is a residually finite T-group (cf. also Residually-finite group);

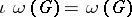unless the subgroup generated by all the finite solvable subnormal subgroups of 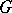is Prüfer-by-finite;

if 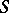is Prüfer-by-finite and infinite, then is metAbelian;

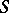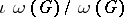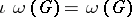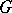if is finite, so is . Finally, if is a finitely generated infinite solvable group.

References

[a1]	R. Baer, "Der Kern, eine charakteristische Untergruppe" Compositio Math. , 1 (1934) pp. 254–283
[a2]	J.C. Beidleman, M.R. Dixon, D.J.S. Robinson, "The generalized Wielandt subgroup of a group" Canad. J. Math. , 47 : 2 (1995) pp. 246–261
[a3]	R. Brandl, S. Franciosi, F. de Giovanni, "On the Wielandt subgroup of infinite soluble groups" Glasgow Math. J. , 32 (1990) pp. 121–125
[a4]	R.A. Bryce, J. Cossey, "The Wielandt subgroup of a finite soluble group" J. London Math. Soc. (2) , 40 (1989) pp. 244–256
[a5]	A.R. Camina, "The Wielandt length of finite groups" J. Algebra , 15 (1970) pp. 142–148
[a6]	C. Casolo, "Soluble groups with finite Wielandt length" Glasgow Math. J. , 31 (1989) pp. 329–334
[a7]	J. Cossey, "The Wielandt subgroup of a polycyclic group" Glasgow Math. J. , 33 (1991) pp. 231–234
[a8]	J. Cossey, "Finite groups generated by subnormal T-subgroups" Glasgow Math. J. , 37 (1995) pp. 363–371
[a9]	F. de Giovanni, S. Franciosi, "Groups in which every infinite subnormal subgroup is normal" J. Algebra , 96 (1985) pp. 566–580
[a10]	H. Heineken, "Groups with restrictions on their infinite subnormal subgroups" Proc. Edinburgh Math. Soc. , 31 (1988) pp. 231–241
[a11]	O.H. Kegel, "Über den Normalisator von subnormalen und erreichbaren Untergruppen" Math. Ann. , 163 (1966) pp. 248–258
[a12]	J.C. Lennox, S.E. Stonehewer, "Subnormal subgroups of groups" , Oxford (1987)
[a13]	E. Ormerod, "The Wielandt subgroup of a metacyclic 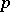-group" Bull. Austral. Math. Soc. , 42 (1990) pp. 499–510
[a14]	D.J.S. Robinson, "Groups in which normality is a transitive relation" Proc. Cambridge Philos. Soc. , 60 (1964) pp. 21–38
[a15]	D.J.S. Robinson, "On the theory of subnormal subgroups" Math. Z. , 89 (1965) pp. 30–51
[a16]	J.E. Roseblade, "On certain subnormal coalition classes" J. Algebra , 1 (1964) pp. 132–138
[a17]	E. Schenkman, "On the norm of a group" Illinois J. Math. , 4 (1960) pp. 150–152
[a18]	H. Wielandt, "Über den Normalisator der subnormalen Untergruppen" Math. Z. , 69 (1958) pp. 463–465