Fatou extension
of a commutative ring 
A commutative ring  containing
containing 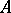 such that each formal power series
such that each formal power series  which is
which is  -rational is in fact
-rational is in fact 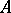 -rational. Recall that a formal power series
-rational. Recall that a formal power series 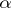 is
is 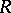 -rational,
-rational, 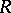 a commutative ring, if there exist two polynomials
a commutative ring, if there exist two polynomials 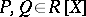 such that
such that 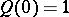 and
and  , that is,
, that is, 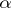 is equal to the formal expansion of
is equal to the formal expansion of 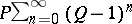 . For instance, if
. For instance, if 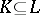 is a field extension (cf. also Extension of a field), then
is a field extension (cf. also Extension of a field), then 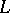 is a Fatou extension of
is a Fatou extension of 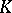 .
.
Fatou extensions are well characterized in the integral case. Thus, from now on, 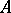 is supposed to be an integral domain with quotient field
is supposed to be an integral domain with quotient field 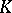 . The example above shows that an integral domain
. The example above shows that an integral domain 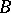 containing
containing 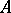 is a Fatou extension of
is a Fatou extension of 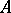 if and only if the ring
if and only if the ring  is a Fatou extension of
is a Fatou extension of 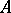 . If the integral domain
. If the integral domain 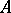 is Noetherian (cf. Noetherian ring), then its quotient field
is Noetherian (cf. Noetherian ring), then its quotient field 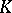 is a Fatou extension of
is a Fatou extension of 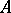 , and, hence, every integral domain containing
, and, hence, every integral domain containing 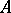 is a Fatou extension of
is a Fatou extension of 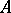 . Many rings are Noetherian: for instance, every finitely generated
. Many rings are Noetherian: for instance, every finitely generated  -algebra is Noetherian.
-algebra is Noetherian.
For a rational function 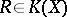 , there are several representations of the form
, there are several representations of the form 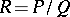 with
with 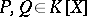 . Such a representation is said to be:
. Such a representation is said to be:
a) unitary if the non-zero coefficient of 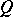 corresponding to the lowest degree is
corresponding to the lowest degree is  ;
;
b) irreducible if 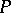 and
and 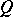 are relatively prime in
are relatively prime in 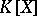 (cf. also Mutually-prime numbers);
(cf. also Mutually-prime numbers);
c) with coefficients in 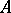 if
if 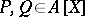 . Let
. Let 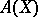 denote the set of rational functions with a unitary representation with coefficients in
denote the set of rational functions with a unitary representation with coefficients in  , and let
, and let 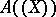 denote the set of Laurent power series, that is,
denote the set of Laurent power series, that is,
 |
(these notations extend the classical notations  and
and 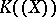 ).
).
To say that the integral domain 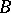 is a Fatou extension of
is a Fatou extension of 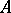 is nothing else than to write:
is nothing else than to write:
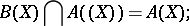 |
in other words, each rational function 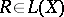 , where
, where 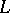 denotes the quotient field of
denotes the quotient field of 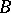 , which has a unitary representation with coefficients in
, which has a unitary representation with coefficients in 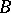 and a Laurent expansion at
and a Laurent expansion at  with coefficients in
with coefficients in 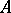 , has a unitary representation with coefficients in
, has a unitary representation with coefficients in 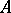 .
.
A rational function 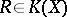 has a unique unitary and irreducible representation. With respect to this representation, there are two main results:
has a unique unitary and irreducible representation. With respect to this representation, there are two main results:
1) The ring 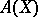 is the set of elements of
is the set of elements of 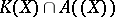 which admit a unitary and irreducible representation whose coefficients are integral over
which admit a unitary and irreducible representation whose coefficients are integral over 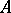 .
.
2) For every element of 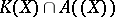 , the coefficients of the unitary and irreducible representation are almost integral over
, the coefficients of the unitary and irreducible representation are almost integral over  . Recall that an element
. Recall that an element  of
of 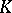 is almost integral over
is almost integral over  if there exists a non-zero element
if there exists a non-zero element  of
of 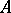 such that
such that 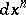 belongs to
belongs to  for each positive integer
for each positive integer  . Each element of
. Each element of 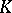 which is integral over
which is integral over 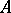 is almost integral over
is almost integral over 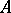 .
.
An integral domain  containing
containing 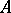 is a Fatou extension of
is a Fatou extension of 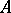 if and only if each element of
if and only if each element of  which is both integral over
which is both integral over 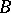 and almost integral over
and almost integral over 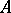 is integral over
is integral over 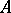 [a1]. The Noetherian case considered above follows from the fact that if
[a1]. The Noetherian case considered above follows from the fact that if 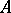 is Noetherian, then each element of
is Noetherian, then each element of 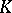 which is almost integral over
which is almost integral over 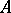 is integral over
is integral over  .
.
The definition of Fatou extension may be easily extended to semi-ring extensions. Then, 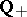 is a Fatou extension of
is a Fatou extension of 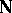 , while
, while 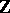 is not a Fatou extension of
is not a Fatou extension of  , nor
, nor 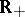 of
of  [a2].
[a2].
Moreover, the notion may be considered for formal power series in non-commuting variables, which have applications in system and control theory [a3]. It turns out that the previous characterization in the integral case still holds.
References
| [a1] | P.-J. Cahen, J.-L. Chabert, "Eléments quasi-entiers et extensions de Fatou" J. Algebra , 36 (1975) pp. 185–192 |
| [a2] | J. Berstel, C. Reutenauer, "Rational series and their languages" , Springer (1988) |
| [a3] | A. Salomaa, M. Soittola, "Automata-theoretic aspects of formal power series" , Springer (1978) |
Fatou extension. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Fatou_extension&oldid=50188