Skorokhod equation
Skorohod equation
A stochastic equation describing a reflecting Brownian motion. Given a one-dimensional Brownian motion  on
on 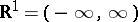 , the reflecting Brownian motion
, the reflecting Brownian motion 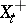 is defined by
is defined by
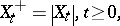 |
which is a Markov process on 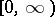 with continuous sample paths.
with continuous sample paths.
A.V. Skorokhod discovered that the reflecting Brownian motion 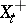 ,
, 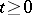 , is identical in law with the solution
, is identical in law with the solution 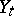 ,
,  , of the equation
, of the equation
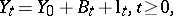 |
where the triple  is a system of real continuous stochastic processes (cf. also Stochastic process) required to have the following properties:
is a system of real continuous stochastic processes (cf. also Stochastic process) required to have the following properties:
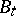 is a one-dimensional Brownian motion starting at
is a one-dimensional Brownian motion starting at  and independent of
and independent of  ;
;
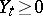 for all
for all 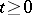 ;
;
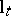 is increasing in
is increasing in 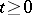 with
with  and
and 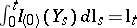 .
.
In fact, the solution 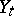 of this Skorokhod equation can be described uniquely and deterministically by the given Brownian motion
of this Skorokhod equation can be described uniquely and deterministically by the given Brownian motion 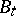 as
as
 |
a formula due to P. Lévy in case that  . Further,
. Further,  is twice the Lévy local time of
is twice the Lévy local time of 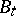 at the origin.
at the origin.
The Skorokhod equation has been extended to the higher-dimensional case 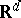 ,
,  , to describe a normally reflecting Brownian motion
, to describe a normally reflecting Brownian motion 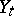 on the closure
on the closure  of a domain
of a domain  . In this case, the equation takes the form
. In this case, the equation takes the form
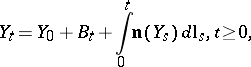 |
where 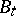 is a
is a 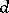 -dimensional Brownian motion starting at the origin,
-dimensional Brownian motion starting at the origin, 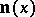 ,
,  , is the inward normal vector field on the boundary
, is the inward normal vector field on the boundary  and
and  is a real increasing process such that
is a real increasing process such that 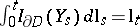 ,
, 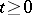 . The third term at the right-hand side of the equation expresses a singular drift, keeping the process
. The third term at the right-hand side of the equation expresses a singular drift, keeping the process 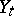 inside
inside 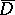 against the isotropic nature of the Brownian motion
against the isotropic nature of the Brownian motion 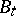 . For a bounded convex domain in
. For a bounded convex domain in 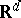 the Skorokhod equation has a unique solution. For other domains, the Skorokhod equations are studied not only from the point of view of stochastic differential equations, but also in relation to other principles, e.g. submartingale problems or Dirichlet forms. Obliquely reflecting Brownian motions, where the vector fields
the Skorokhod equation has a unique solution. For other domains, the Skorokhod equations are studied not only from the point of view of stochastic differential equations, but also in relation to other principles, e.g. submartingale problems or Dirichlet forms. Obliquely reflecting Brownian motions, where the vector fields  in the Skorokhod equations are different from the normal vector field, also arise naturally in the diffusion approximation in stochastic network theory.
in the Skorokhod equations are different from the normal vector field, also arise naturally in the diffusion approximation in stochastic network theory.
References
| [a1] | N. Ikeda, S. Watanabe, "Stochastic differential equations and diffusions" , North-Holland (1989) (Edition: Second) |
Skorokhod equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Skorokhod_equation&oldid=50138