Lyapunov equation

Usually, the matrix equation

(a1)

where the star denotes transposition for matrices with real entries and transposition and complex conjugation for matrices with complex entries; 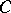is symmetric (or Hermitian in the complex case; cf. Hermitian matrix; Symmetric matrix). In fact, this is a special case of the matrix Sylvester equation

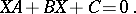(a2)

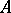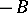The main result concerning the Sylvester equation is the following: If and have no common eigenvalues, then the Sylvester equation has a unique solution for any .

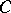When 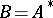and there are no eigenvalues 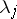of 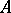such that 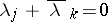whatever 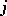and 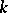are (in the numbering of eigenvalues of 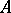), then (a1) has a unique Hermitian solution for any . Moreover if 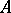is a Hurwitz matrix (i.e. having all its eigenvalues in the left half-plane, thus having strictly negative real parts), then this unique solution is

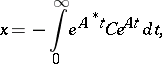(a3)

and if 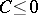, then 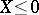. From this one may deduce that if 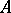and 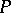satisfy 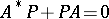, than a necessary and sufficient condition for to be a Hurwitz matrix is that 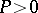. In fact, this last property justifies the assignment of Lyapunov's name to (a1); in Lyapunov's famous monograph [a1], Chap. 20, Thm. 2, one finds the following result: Consider the partial differential equation

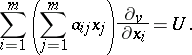(a4)

If 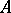has eigenvalues with strictly negative real parts and 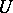is a form of definite sign and even degree, then the solution, 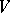, of this equation will be a form of the same degree that is sign definite (with sign opposite to that of 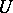. Now, if 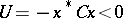with , then 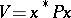, with 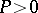, is a solution of (a1). In fact, 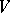is a Lyapunov function for the system

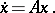(a5)

These facts and results have a straightforward extension to the discrete-time case: for the system

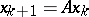(a6)

one may consider the quadratic Lyapunov function as above (i.e. 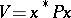) and obtain that 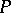has to be a solution of the discrete-time Lyapunov equation

(a7)

whose solution has the form

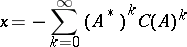(a8)

provided the eigenvalues of 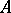are inside the unit disc.

The equation may be defined for the time-varying case also. For the system

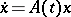(a9)

one may consider the quadratic Lyapunov function 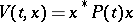and obtain that 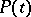has to be the unique solution, bounded on the whole real axis, of the matrix differential equation

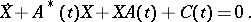(a10)

This solution is

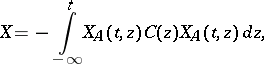(a11)

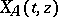being the matrix solution of 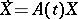, 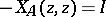. The solution is well defined if 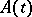defines an exponentially stable evolution (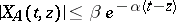, 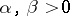). It is worth mentioning that if 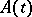and 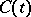are periodic or almost periodic, then defined by (a11) is periodic or almost periodic, respectively. Extensions of this result to a discrete-time or infinite dimensional (operator) case are widely known. Actually, the Lyapunov equation has many applications in stability and control theory; efficient numerical algorithms for solving it are available.

References

[a1]	A.M. Lyapunov, "General problem of stability of motion" , USSR Acad. Publ. House (1950) (In Russian)
[a2]	R.E. Bellman, "Introduction to matrix-analysis" , McGraw-Hill (1960)
[a3]	A. Halanay, "Differential equations: stability, oscillations time lags" , Acad. Press (1966)
[a4]	A. Halanay, D. Wexler, "Qualitative theory of pulse systems" , Nauka (1971) (In Russian)
[a5]	A. Halanay, V. Räsvan, "Applications of Lyapunov methods in stability" , Kluwer Acad. Publ. (1993)