Egorov generalized function algebra
Given an open subset 
of 
, Yu.V. Egorov [a1] defined the generalized function algebra 
as the factor algebra of 
modulo the ideal of sequences 
which vanish eventually on every compact subset of 
. The family 
provides a sheaf of differential algebras on 
. Convolution with a sequence of mollifiers 
, where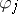 
converges to the Dirac measure, gives an imbedding of the space 
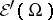of compactly supported distributions into 
which respects derivatives as well as supports. It can be extended as a sheaf morphism to an imbedding of the space of distributions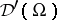 
.
As a generalized function algebra, 
can be employed to study non-linear partial differential equations. In particular, Egorov has used the algebra to construct generalized solutions to boundary value problems as well as evolution equations. In the latter case the spatial derivative 
may be replaced by the difference operator
 |
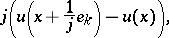where 
denotes the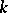 
th unit vector in 
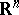. This way partial differential equations are approximated by ordinary difference-differential equations in the algebra 
.
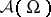See also Generalized function algebras.
References
| [a1] | Yu.V. Egorov, "A contribution to the theory of generalized functions" Russian Math. Surveys , 45 : 5 (1990) pp. 1–49 |
Egorov generalized function algebra. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Egorov_generalized_function_algebra&oldid=49979